DOI: https://doi.org/10.15446/abc.v21n3.54218
PLANKTIC FORAMINIFERAL DIVERSITY: LOGISTIC GROWTH OVERPRINTED BY A VARYING ENVIRONMENT
Diversidad en foraminíferos planctónicos: crecimiento logístico sobrepuesto por un ambiente variable
Andrés L. CÁRDENAS-ROZO1; Peter J. HARRIES2.
1 Ciencias de la Tierra, Escuela de Ciencias, Universidad EAFIT. Cra. 49 n°. 7 Sur-50. Medellín, Colombia.
2 Marine, Earth, and Atmospheric Sciences Faculty, Collegue of Sciences, North Carolina State University. 2800 Faucette Drive, 1125 Jordan Hall, Campus Box 8208. Raleigh, North Carolina, USA.
For correspondence. acarde17@eafit.edu.co
Received: 17th November 2015, Returned for revision: 20th July 2016, Accepted: 2nd August 2016.
Associate Editor: John Charles Donato Rondón.
Citation/Citar este artículo como: Cárdenas-Rozo AL., Harries PJ. Planktic foraminiferal diversity: logistic growth overprinted by a varying environment. Acta biol. Colomb. 2016;21(3):501-508. DOI: https://doi.org/10.15446/abc.v21n3.54218
Este artículo fue presentado por invitación de la Red Colombiana de Biología Evolutiva (COLEVOL) y la revista Acta Biológica Colombiana con el fin de incentivar la investigación en el área de biología evolutiva.
ABSTRACT
This study statistically assesses the relationship between the planktic foraminiferal long-term diversity pattern (~170 Ma to Recent) and four major paleobiological diversification models: (i) the 'Red Queen' (Van Valen, 1973; Raup et al., 1973), (ii) the turnover-pulse (Vrba, 1985; Brett and Baird, 1995), (iii) the diversity-equilibrium (Sepkoski, 1978; Rosenzweig, 1995), and (iv) the 'complicated logistic growth' (Alroy, 2010a). Our results suggest that the long-term standing diversity pattern and the interplay between origination and extinction rates displayed by this group do not correspond to the first three models, but can be more readily explained by the fourth scenario. Consequently, these patterns are likely controlled by a combination of planktic foraminiferal interspecific competition as well as various environmental changes such as marine global temperatures that could impacted the niches within the upper mixed layer within the oceans. Moreover, as other global long-term patterns have been interpreted as reflecting 'complicated logistic growth', this study further suggests that the interplay between abiotic and biotic factors are fundamental elements influencing the evolutionary processes over the extensive history of the biota.
Keywords: abiotic and biotic controls, complicated logistic growth, diversity dynamics, macroevolution, planktic foraminifera, paleobiology.
RESUMEN
Este estudio evalúa estadísticamente la relación entre el patrón de diversidad global de los foraminíferos planctónicos en el largo plazo (~170 Ma al Reciente) y los cuatro modelos de diversificación propuestos desde la rama de la paleobiología: (i) "Reina Roja" (Van Valen, 1973; Raup et al., 1973), (ii) remplazo pausado (Vrba, 1985; Brett y Baird, 1995), (iii) diversidad en equilibrio (Sepkoski, 1978; Rosenzweig, 1995), y (iv) el "crecimiento logístico complicado" (Alroy, 2010a). Nuestros resultados sugieren que la forma de este patrón global de diversidad y la inter-relación entre las tasas de extinción y originación de este grupo no corresponden con los primeros tres modelos anteriormente citados. Sin embargo, estos pueden ser explicados bajo el cuarto escenario. Consecuentemente, las dinámicas de diversidad (i.e. patrón de diversidad y tasas de extinción y originación) de este grupo posiblemente son controladas por la combinación de la competencia interespecífica de los foraminíferos planctónicos y varios cambios ambientales tales como temperaturas globales marinas que pudieron impactar el número de nichos dentro de la capa superior de los océanos. Además, otros patrones globales de diversidad en el largo plazo han sido interpretados como el reflejo del modelo de crecimiento logístico complicado, lo que sugiere que la relación entre factores abióticos y bióticos tiene un carácter fundamental en los procesos evolutivos que han sucedido a lo largo de la historia de la vida.
Palabras clave: crecimiento logístico complicado, controles abióticos y bióticos, dinámicas de diversidad, foraminíferos planctónicos, macroevolución, paleobiología.
INTRODUCTION
Planktic foraminifers, a group of extant sexually reproducing protists, have been an extremely useful group for testing possible controls on long-term diversity patterns (e.g., Cifelli,1969; Frerichs, 1971; Lipps, 1979; Wei and Kennett, 1986; Schmidt et al., 2004; Ezard et al., 2011; Peters et al., 2013). In part, this is a reflection of their extremely wide biogeographic distribution and the fact that virtually continuous microfossil successions can be accessed through the study of outcrops and especially deep-sea cores dating back to the Bajocian (~170 Ma). Despite the interest in this group, only a few studies have examined possible controls on their long-term diversity dynamics using quantitative approaches (e.g., Wei and Kennett, 1986; Schmidt et al., 2004; Ezard et al., 2011), and these studies have been concentrated on specific time intervals rather than on the group's entire fossil history (e.g., Neogene [~23 Ma to Recent; Wei and Kennett, 1986] and Cenozoic [~65.5 Ma to Recent; Schmidt et al., 2004 and Ezard et al., 2011]). Moreover, even though comprehensive models have been developed to explain underlying long-term diversity dynamics, these likely need to combine 'abiotic' and 'biotic' elements rather to contrast them (Alroy, 2010a); to date only Ezard's et al., (2011) work has employed that approach in examining the role of changing oceanic temperatures and species' ecologies as controls on the Cenozoic diversity pattern.
Consequently, this study aims to augment and extend this approach by examining planktic foraminifer diversity dynamics across the group's entire fossil history (~170 to 0 Ma) and to analytically describe their long-term diversity pattern through the use of an integrated abiotic-biotic model. This approach involves the comparison of quantitative turnover predictions of each one of the four major paleobiological diversification models: (i) the 'Red Queen' scenario (Van Valen 1973; Raup et al., 1973); (ii) the turnover-pulse model (Vrba, 1985; Brett and Baird, 1995); (iii) the diversity-equilibrium model (Sepkoski, 1978; Rosenzweig, 1995); and (iv) the 'complicated logistic growth' scenario, where diversity limits are imposed by variable extrinsic factors (Alroy, 2010a). The fundamental basis of this approach is to employ time-series analyses of changes in global planktic foraminiferal diversity dynamics (i.e., the relationships between extinction and origination rates as well as between those rates and diversity), and, mirroring previous studies, testing between changes in both global long-term planktic foraminiferal diversity and mean global marine temperature (i.e., changes in the oceanic upper mixed layer as influenced by temperature variation, represents an important control on global long-term diversity standing pattern for the planktic foraminifers over the long-term; Peters et al., 2013).
MATERIALS AND METHODS
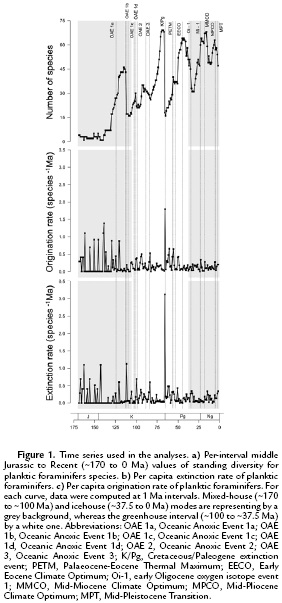
This study is based on a compilation of 699 planktonic foraminifera species' stratigraphic ranges from published sources spanning the Middle Bajocian (~ 170 Ma) to Recent, calibrated to Gradstein et al., (2012) geologic timescale, and binned into 1 Ma intervals (see raw data in Appendix; Cárdenas, 2012). These stratigraphic ranges were used to determine standing diversity, extinction and origination rates. Furthermore, these data were then analyzed using both the boundary-crossing (BC; Bambach, 1999) and per-capita (Foote, 2000) methodologies, respectively (Fig. 1). The BC approach was preferred in the computation of planktic foraminifers diversity curve as opposed to subsampling procedures (i.e., sampling in bin and shareholder quorum; Alroy, 2010b), primarily because these other methodologies require information related to individual taxonomic occurrences (i.e., abundances), and, even though these kind of data are available for planktic foraminifers in numerous biostratigraphic reports from the Deep Sea Drilling Project/Ocean Drilling Project, they are primarily from the Late Cretaceous to Recent interval. Therefore, an application of this procedure would result in the loss of 60 % of the planktic foraminfers' total temporal longevity (i.e., ~170 to ~70 Ma would be excluded). In the same way, given that the raw data for the computation of extinction and origination rates was based on first and last appearances of the taxa, the per-capita-rates technique for calculating extinction and origination rates (Foote, 2000) was selected over newer techniques, such as the 'three-timers' approach, as the latter necessitates a fossil-occurrence database for the calculations (Alroy, 2010b); such a database does not currently exist for the Foraminifera. Additionally, because not all taxa present during a given 1 Ma bin ranged throughout the entire interval, the total diversity of an interval likely overestimates the number of species at extinction risk at any given time. Per-capita rates were used as they address those sorts of biases by drawing a census of taxa at precise moments in time (i.e., the beginning and the end of the interval) (Foote, 2000; Foote and Miller, 2007). Finally, to avoid spurious correlations resulting from serial auto-correlation and 'noisy' secular trends (McKinney and Oyen, 1989), the datasets were analyzed using first-generalized differences before the use of the statistical correlation to compare turnover rates (i.e., extinction versus origination) both to each other as well as with the standing diversity pattern. Owing to the non-normal character of those variables throughout the planktic foraminifers' fossil history (Appendix), Spearman's rank-order coefficient was used to test for correlation of changes in both the extinction and origination per-capita rates, as well as changes in species richness and in turnover rates (i.e., extinction and origination). Furthermore, we tested whether cross-correlations among planktic foraminiferal diversity dynamics time series (i.e., diversity pattern and extinction-origination per-capita rates) varied when binned into different global climatic mode intervals, comprising a 'mixed house' interval characterized by evidence for both warm (e.g., Littler et al., 2011) and cool temperatures (e.g., Frakes et al., 1992; Veizer et al., 2000) (~170 to ~100 Ma; Bajocian-Albian), a greenhouse phase (~100 to ~37.5 Ma; Cenomanian-late early Eocene; Miller et al., 2005), and an icehouse phase (~37.5 to 0 Ma; latest Eocene-Recent; Miller et al., 2005) (Fig. 1)). Finally, a Kolmogorov-Smirnov test for equality of distribution was employed to perform a statistical comparison of the complete distribution frequency shapes of both 'turnover-pulse' model (Appendix) and planktic foraminiferal first and last occurrence data (Appendix).
RESULTS
The computed global planktic foraminiferal diversity pattern showed that species richness remained very low initially and then increased ~30 Ma after the initial appearance of the clade (Fig. 1). Furthermore, the data also show that apart from major turnover events forced by significant global environmental events (i.e., at the Aptian-Albian, Cretaceous-Paleogene, and Eocene-Oligocene boundaries), it has 'lesser' troughs during the mid-Cretaceous, mid-Paleogene, and mid-Neogene. Moreover, the planktic foraminiferal diversity's general trend has not been neither constantly increasing nor reaching a saturation level (Fig. 1). However, extinction and origination per-capita rates through the clade's history have their highest peaks during the group's earliest evolutionary phase (~170 Ma to 145 Ma) and surrounding the Cretaceous-Paleogene boundary (Fig. 1). Furthermore, the extinction and origination per-capita rates had values of zero only during the first 30 Ma of the group's evolutionary history (Fig. 1, Appendix).
Spearman's rank-order correlation test applied to the first-generalized differences in both planktic foraminiferal extinction and origination per-capita rates throughout the fossil history of the group (170-0 Ma), reveal a significant positive association (ρ = 0.473, p = 1.07*10-10) (Fig. 2). Furthermore, using an identical statistical approach over the same interval, the correlation between planktic foraminiferal standing diversity display a significant relationship when compared to both extinction and origination per-capita rates (ρ = -0.245, p = 1.42*10-3 and ρ = 0.384, p = 2.96*10-7, respectively). Additionally, when these variables were binned relative to the dominant global climatic modes, significant negative and positive associations between diversity-extinction and diversity-origination regressions, respectively, were also found (Table 1). Moreover, when Spearman's rank-order test was used in evaluating variation in both extinction and origination per-capita rates with future changes in diversity and changes in extinction per-capita rates with future changes in origination per-capita rates, both tested by offsetting richness and evolutionary rates by one bin (= 1 Ma), no significant relationships remain (Table 2). Finally, the Kolmogorov-Smirnov test of the equality of frequency distribution displayed a significant difference among the overall shapes of planktic foraminifers' first appearance and last appearance distributions (D = 0.1001 p = 1.80*10-3; Fig. 3).
DISCUSSION
During the first ~30 Ma of planktic foraminiferal evolutionary history, the computed standing diversity has a relatively invariant character in terms of overall species richness with an average value of 2.61 species (CI: 2.25 to 2.96, computed after the 95 % confidence intervals bootstrapped 1000 times), whereas both extinction and origination per-capita rates display a very volatile pattern, characterized by several peaks of varying magnitude but also troughs in which both per-capita rates are zero (Fig. 1, Appendix). In part, this volatility is a function of the very low species richness during this interval such that even the origination or extinction of a single species can have a disproportionate impact as compared to later in the group's history.
In addition, this may also potentially be a result of lower planktic foraminiferal interspecific competition levels (Table 1; the value of extinction-origination cross-correlation is lower during the mixed-house interval as compared to the other two stages) associated with the initial invasion of the upper water column due to the evolutionary innovation of a 'free-floating' life habit from a benthic progenitor (Boudagher et al., 1997). This adaptive radiation allowed the expansion of the foraminifers' habitat into a previously unoccupied ecological domain where the lack of constraints likely allowed evolutionary experimentation.
Following that phase, the standing-diversity curve dramatically increases, but rather than increasing exponentially or eventually reaching a plateau, the curve displays several peaks and troughs, which not only coincide with global events that disrupted the biota (i.e, Aptian-Albian bioevent as well as the Cretaceous-Paleogene and Eocene-Oligocene mass extinctions; Fig. 1), suggesting that changes in global diversity for this group, could also be related to a different set of environmental/paleobiological alterations (e.g. changes in niche availability, changes in types of competition). On the other hand, the turnover rates display a diminished intensity in their peaks from the Late Mesozoic through the Cenozoic (with exception of the values associated with the Cretaceous-Paleogene boundary), and even though there are various lower values, as reflected in the troughs, they are less prominent than during the initial ~30 Ma of the group during which the minimum values for both per-capita extinction and origination rates exceed zero (Fig. 1, Appendix, see online). Moreover, through time there is an increase in the per-capita extinction-origination cross-correlation values (Table 1). Lower values of extinction-origination cross-correlation occurred during the mixed-house interval, increased during the greenhouse stage, and are even higher through the icehouse period. In part, this continuous strengthening of the correlation between these two components likely suggests an intensification of planktic foraminiferal interspecific competition given that species have acquired a higher fitness in the pelagic environment and/or the enhancement of niche partitioning into a more densely inhabited ecological space.
The 'stationary' models of diversification (i.e., 'turnover pulse' [Vrba 1985] and 'coordinated stasis' [Brett and Baird, 1995]) necessitate that extinction and origination rates be cross-correlated to balance or offset any changes (Alroy, 1996; Alroy, 2008). Furthermore, these two models also predict no significant difference between the frequency distribution of the first and last appearance data given that long intervals (i.e., millions of years) of evolutionary stasis, resulting from a relatively consistent set of extrinsic environmental conditions (Vrba, 1985) and/or strong stabilizing selection as postulated by Brett and Baird (1995), must be separated by virtually instantaneous (from a geological perspective of time) evolutionary pulses induced by major environmental shifts, allowing an increase in environmental fragmentation (Vrba, 1985) and/or accompanying biotic reorganizations (Brett and Baird 1995). Results obtained here, despite the Spearman-rank-order tests displaying a positive correlation between origination and extinction per-capita rates throughout the entire planktic foraminifers fossil history, do not support these two diversification hypotheses, as there is no correlation between origination and extinction per-capita rates when offsetting is performed (Table 2) and a significant difference in the equality of distribution between the curves of first and last appearances throughout the groups fossil history (~170 Ma to Recent; Fig. 3) as tested using the Kolmogorov-Smirnov test. Consequently, this lack of coordination between these two variables does not support the notion that long-term intervals of morphological/evolutionary inertia within the planktic foraminifera shifted only when disrupted by environmental events.
Another oft-cited diversification hypothesis is the Red Queen model which postulates that the diversification pattern through time has been shaped by various competitive interactions between taxa (e.g. competition for resources, predator-prey interactions) leading to an unending escalation of adaptations (Van Valen, 1973). A major prediction based on this hypothesis is that diversity should grow exponentially through time, given that the constant turnover rates would be driven by biotic interactions and be, therefore, immune to environmental forcing (Van Valen, 1973; Benton, 2009; Alroy, 2010a). According to this criterion, the analysis of planktic foraminiferal diversity dynamics applied in this study do not support the Red Queen's predictions as the diversity pattern does not follow an exponential pattern (Kolmogorov-Smirnov test for equality of distribution between planktic foraminifera empirical diversity (Fig. 3a) and a simulated planktic foraminifera exponential diversity growth (Fig. 3b) showed a significant difference in distribution between the two curves: p = 2.2*10-16), and both extinction and origination per-capita rates (Fig. 1) show a significant negative trend through time (Spearman-rank-order results after the regression of extinction per-capita rates against time: ρ = -0.269, p = 4.25*10-4, Spearman-rank-order results after the regression of origination per-capita rates against time: ρ = -0.209, p = 6.61*10-4).
An alternative explanation of long-term diversification is the so-called 'diversity equilibrium' model (Sepkoski, 1978; Rosenzweig, 1995; Alroy, 2008). Based on this, long-term diversification processes should obtain an equilibrium level at some point as a result of the following turnover-relationship scenarios: (i) a secular increase and decline in extinction and in origination rates, respectively (Gilinsky and Bambach, 1987), (ii) the existence of a positive cross-correlation between extinction rates with diversity levels and/or a negative cross-correlation among origination rates and standing diversity (i.e., density dependence of rates; Sepkoski, 1978; Rosenzweig, 1995), and (iii) a positive cross-correlation between extinction and origination rates given that origination pulses will respond to bursts of extinction and as well as vice versa (Webb, 1969; Mark and Flessa, 1977).
The current results do not support the first two components of this model given the significant negative trends through time for both extinction and origination per-capita rates (ρ = -0.269, p = 4.25*10-4 and ρ = -0.209, p = 6.60*10-3), and the significant, although opposite to the postulated relationship (i.e., positive relationship between origination and standing diversity and negative correlation between extinction and species richness), cross-correlations between changes in both extinction and origination per-capita rates with changes in diversity throughout time. Nevertheless, the presence of a significant positive cross-correlation between origination and extinction per-capita rates suggests that the long-term planktic foraminiferal diversity pattern could be described by a logistic growth pattern with no extrinsic controls and limited only by intrinsic ecological factors (i.e., planktic foraminiferal interspecific competition) as proposed by Webb (1969) and Mark and Flessa (1977). However, the overall logistic growth pattern predicted by this model is not displayed by the planktic foraminifers standing diversity (Fig. 1). Rather than gradually approaching an equilibrium level, the curve is instead characterized by several peaks and troughs, in addition to those reflecting elevated turnover forced by major biotic disruptions (i.e., Aptian-Albian, Cretaceous-Paleogene, Eocene-Oligocene). Given that the data do not match the predicted response, this hypothesis is also rejected for planktic foraminifers.
A final diversification scenario to be considered is the 'complicated logistic growth', which predicts that diversity limits are imposed by changing extrinsic factor(s) (Alroy, 2010a). As predicted by this model, diversity should reach a carrying capacity that in turn is controlled by varying external components (e.g., niche availability, resource abundance, richness and abundance of an interacting group, specifically either prey or predators). As a result, the diversity pattern predicted by this model does not mirror a logistic curve, instead it should display peaks and troughs suggesting a dynamic record being driven by medium- to long-term shifts in the carrying capacity forced by various external factors (Sepkoski, 1984; Alroy, 2010a). Moreover, competition should be sufficiently strong to maintain a level of diversity close to its saturation point in response to any environmental shifts, and the extrinsic factor(s) should change through time; if the extrinsic factor(s) does/do not change, the diversity pattern should 'plateau' (Alroy, 2010a). Consequently, the combination of the lack of a logistic standing diversity pattern (Fig. 1), the positive cross-correlation between changes in extinction and origination per-capita rates indicating significant foraminiferal levels of competition through all the fossil history of the group, and the significant association between changes in planktic foraminifers diversity pattern with various extrinsic environmental factor (e.g., changes in marine global temperature, as suggested by Schmidt et al., 2004; Peters et al., 2013; Frass et al., 2015), all indicate that the planktic foraminifers diversity pattern approaches logistic growth over relatively short intervals, due to fluctuating diversity limits imposed by changing extrinsic factor(s) that overprint that pattern. Furthermore, the 'non-equilibrium' state of global planktic foraminifers diversity through time is likely a result of continual changes in environmental conditions which influenced niche availability (e.g., Rutherford et al., 1999). This produces a diversity trend which, rather than equilibrating at a given level and producing a plateau, is instead highly dynamic and variable with diversity increasing and decreasing as upper mixed-layer niches expand and contract, respectively. This is supported by the results obtained here which display both significant cross-correlations between extinction per-capita rates and standing diversity (negative) as well as between origination per-capita rates with standing diversity (positive).
CONCLUSIONS
The quantitative examination of intrinsic planktic foraminifers' diversity dynamics throughout their entire fossil history (~170 Ma to Recent) supports the interpretation that this group's long-term diversity pattern follows the 'complicated logistic growth' model, which has also been documented for the global marine Phanerozoic record of macrofossils (Alroy, 2010a). As proposed by Rosenzweig (1995), this indicate that diversity is a (i) self-regulating property of natural systems, with a dynamic eroding and restoring of the number of taxa, and (ii) does not increase infinitely through vast amounts of time given the evolutionary limitations imposed by various extrinsic components. For planktic foraminifers, diversity changes are likely controlled by niche availability, which is a function of both diversity as well as various biologic and environmental factors. Consequently, this long-term diversity pattern likely has changed in lockstep with the vertical range of the pelagic upper-mixed layer. These changes possibly influenced available niche space and potentially the overall number of niches. Furthermore, given that planktic foraminifer interspecific competition, as inferred by the significant cross-correlation displayed by changes in both extinction and origination per-capita rates, has been sufficiently strong to track the carrying capacity of the system (forced by the global oceanic temperature changes) through time, the 'complicated logistic growth' long-term diversification scenario is the most parsimonious model explaining the shape of the global long-term planktic foraminifers diversity pattern. Finally, our study strongly suggests that a full understanding of long-term diversity patterns must be rooted in the analysis of both intrinsic and extrinsic biotic and abiotic controls.
Appendix data of this manuscript are available at Acta Biológica Colombiana Online version.
AKNOWLEDGEMENTS
We thank J.I. Martínez for the insightful comments on an earlier draft, and the two anonymous reviewers. This research was partially supported by research grants from Sigma Xi G200803150409, the Paleontological Society, and the Geological Society of America.
REFERENCES
Alroy J. Constant extinction, constrained diversification, and uncoordinated stasis in North American mammals. Palaeogeogr Palaeoclimatol Palaeoecol. 1996;127(1):285-311. Doi:10.1016/S0031-0182(96)00100-9.
Alroy J. Dynamics of origination and extinction in the marine fossil record. Proc Natl Acad Sci USA. 2008;105(1):11536-11542. Doi:10.1073/pnas.0802597105.
Alroy J. Geographical, environmental and intrinsic biotic controls on Phanerozoic marine diversification. Palaeontology. 2010a;53(6):1211-1235. Doi:10.1111/j.1475-4983.2010.01011.x.
Alroy J. Fair sampling of taxonomic richness and unbiased estimation of origination and extinction rates. In: Alroy J, Hunt G, editors. Quantitative Methods in Paleobiology. Boulder: The Paleontological Society; 2010b. p. 55-80.
Bambach RK. Energetics in the global marine fauna: a connection between terrestrial diversification and change in the marine biosphere. Geobios. 1999;32(2):131-144. Doi: 10.1016/S0016-6995(99)80025-4.
Barnosky AD. Distinguishing the effects of the Red Queen and Court Jester on Miocene mammal evolution in the northern Rocky Mountains. J Vert Pal. 2001;21(1):172-185. Doi: 10.1671/0272-4634(2001)021[0172:DTEOTR]2.0.CO;2.
Benton M J. The Red Queen and the Court Jester: Species diversity and the role of biotic and abiotic factors through time. Science. 2009;323(5915):728-732. Doi:10.1126/science.1157719.
Brett CE, Baird GC. Coordinated stasis and evolutionary ecology of Silurian to Middle Devonian faunas in the Appalachian Basin. In: Erwin DH, Anstey RL. editors. New approaches to speciation in the fossil record. New York City: Columbia University Press; 1995. p. 285-315.
Boudagher MK, Banner FT, Whittaker JE. The Early Evolutionary History of Planktonic Foraminifera. London: Springer; 1997. 288 p.
Cárdenas AL. Paleobiological assessment of controls underlying long-term diversity dynamics (Doctoral dissertation). Florida (USA): School of Geosciences, University of South Florida; 2012. 272 p.
Cifelli R. Radiation of Cenozoic planktonic foraminifera. Syst Zool. 1969:18:154-168. Doi:10.2307/2412601.
Ezard TH, Aze T, Pearson PN, Purvis A. Interplay between changing climate and species' ecology drives macroevolutionary dynamics. Science. 2011;332(6027):349-351. Doi:10.1126/science.1203060.
Foote M. Origination and extinction components of taxonomic diversity: general problems. Paleobiology. 2000;26(sp4):74-102. Doi: https://doi.org/10.1666/0094-8373(2000)26[74:OAECOT]2.0.CO;2.
Foote M, Miller AI. Principles of Paleontology. New York: Freeman; 2007. 480 p.
Frass AJ, Clay Kelly D, Peters SE. Macroevolutionary history of the planktic foraminifera. Annu Rev Earth Planet Sci. 2015;43(5.1):5-28. Doi:10.1146/annurev-earth-060614-105059.
Frakes LA, Francis JE, Syktus JI. Climate Modes of the Phanerozoic. New York: Cambridge University Press; 1992. 288 p.
Frerichs WE. Evolution of planktonic foraminifera and paleotemperatures. J Paleo. 1971;45(6):963-968.
Gradstein FM, Ogg JG, Schmitz AG, Ogg G. A geologic time scale 2012. Walthman: Elsevier; 2012. 610 p.
Gilinsky NL, Bambach RK. Asymmetrical patterns of origination and extinction in higher taxa. Paleobiology. 1987;13(4):427-445.
Lipps JH. Ecology and paleoecology of planktic foraminifera. In: Lipps JH, Berger WH, Buzas MA, Douglas RG, Ross CA, editors. Foraminiferal Ecology and Paleoecology. Houston: SEPM Short Courses Notes; 1979. p. 62-104.
Littler K, Robinson SA, Brown PR, Nederbragt AJ, Pancost RD. High sea-surface temperatures during the Early Cretaceous epoch. Nat Geosc. 2011;4(3):169-172. Doi:10.1038/ngeo1081.
Mark GA, Flessa KW. A test for evolutionary equilibria: Phanerozoic brachiopods and Cenozoic mammals. Paleobiology. 1977;3(1):17-22.
McKinney ML, Oyen CW. Causation and nonrandomness in biological and geological time series: temperature as a proximal control of extinction and diversity. Palaios. 1989;4(1):3-15.
Peters SE, Kelly DC, Frass AJ. Oceanographic controls on the diversity and extinction of planktonic foraminifera. Nature. 2013;493(7432):398-403. Doi:10.1038/nature11815.
Raup DM, Gould SJ, Schopf TM, Simberloff DS. Stochastic models of phylogeny and the evolution of diversity. J Geol. 1973;81(5):525-542.
Rosenzweig ML. Species diversity in space and time. New York: Cambridge University Press; 1995. 436 p.
Rutherford S, D'Hondt SD, Prell W. Environmental controls on the geographic distribution of zooplankton diversity. Nature. 1999;400(6746):749-753. Doi:10.1038/23449.
Schmidt DN, Thierstein HR, Bollmann J, Schiebel R. Abiotic forcing of plankton evolution in the Cenozoic. Science. 2004;303(5655):207-210. Doi:10.1126/science.1090592.
Sepkoski Jr. JJ. A kinetic model of Phanerozoic taxonomic diversity. I. Analysis of marine orders. Paleobiology. 1978;4(3): 223-251.
Sepkoski Jr. JJ. A kinetic model of Phanerozoic taxonomic diversity. III. Post-Paleozoic families and mass extinctions. Paleobiology. 1984;10(2):246-267.
Van Valen L. A new evolutionary law. Evolutionary Theory. 1973;1(1):1-30.
Veizer J, Godderis Y, Francis LM. Evidence for decoupling of atmospheric CO2 and global climate during the Phanerozoic eon. Nature. 2000;408(6813):698-701. Doi:10.1038/35047044.
Vrba E. Turnover-pulses, the Red Queen, and related topics. Amer J Sci. 1985;293:418-452. Doi:10.2475/ajs.293.A.418.
Webb SD. Extinction-origination equilibria in Late Cenozoic land mammals of North America. Evolution. 1969;23(4):688-702. Doi:10.2307/2406863.
Wei KY, Kennett JP. Taxonomic evolution of Neogene planktonic foraminifera and paleoceanographic relations. Paleoceanography. 1986(1);1:67-84. Doi:10.1029/PA001i001p00067.