
DOI: https://doi.org/10.15446/dyna.v83n198.49766
Transient analysis of mixed wind parks with different turbine types
Análisis de transitorios de parques eólicos mixtos con diferentes tipos de turbinas
Helleson J. B. da Silva a & Carolina M. Affonso a
a Faculty of Electrical Engineering, Federal University of Para, Pará, Brasil. helleson_jorthan@hotmail.com, carolina@ufpa.br
Received: March 23th, 2015. Received in revised form: November 1rd, 2015. Accepted: May 5th, 2016.
This work is licensed under a Creative Commons Attribution-NonCommercial-NoDerivatives 4.0 International License.

Abstract
The
variable-speed wind turbine is widely used in new wind parks and became the
dominant type of wind turbine due to its technological advantages. However, in
the case of already installed wind parks based on fixed speed wind turbines,
the immediate migration from one technology to another becomes economically
impracticable; the process of gradual modernization is more suitable. In this
sense, this paper presents a detailed analysis of a mixed wind park containing
both doubly fed and squirrel cage induction generators. It considers different
rates of capacity for each technology and implementing the gradual replacement
of some fixed speed units by variable speed units. The study addresses aspects
of voltage stability, fault analysis, wind speed variation and critical fault
clearance time. The network used to perform simulations is a real system from
Brazil. The results show that DFIG improves the wind park behavior in many
aspects and can be a cost-effective solution.
Keywords: wind generation; squirrel cage induction generator; doubly fed induction generator; stability.
Resumen
La
turbina eólica de viento variable se volvió el tipo dominante de turbina en
nuevos parques eólicos debido a sus ventajas tecnológicas. En caso de parques
eólicos ya en funcionamiento con turbinas basadas en viento constante, la
migración de una tecnología hacia la otra se vuelve económicamente inviable, lo
que requiere su modernización gradual. En ese sentido, este artículo presenta
un análisis de un parque eólico mixto de generadores de inducción con jaula de
ardilla y doblemente alimentados, simulando el reemplazo gradual de algunas
unidades de velocidad fija por otras de velocidad variable. El estudio abarca
aspectos de estabilidad de tensión, análisis de faltas, variación de la
velocidad del viento y tiempo crítico de eliminación de faltas. El sistema de
potencia empleado es un sistema real brasileño y los resultados muestran que
generadores de inducción doblemente alimentados mejora el desempeño del parque
eólico en distintos aspectos, siendo una solución económicamente efectiva.
Palabras clave: generación eólica; generador de inducción de jaula de ardilla; generador de inducción doblemente alimentado; estabilidad.
1. Introduction
Wind generation is gaining increasing interest around the world today since it can provide a clean, cheap and renewable source of energy for many people. In Brazil, wind is the fastest growing source of power generation with an installed capacity of 6 GW at the end of 2014, representing 4.5% of the country's total installed capacity [1].
There are two main popular wind power technologies in the world: fixed speed turbines and variable speed turbines. The fixed speed turbines use squirrel cage induction generators (SCIG) and have variations in output power, mechanical stress and limited power quality control. Also, they always draw reactive power from the grid and it is necessary to use capacitor banks. The advantage of this scheme is the low cost and robust structure [2].
The variable speed turbines are modern systems and can use doubly fed induction generators (DFIG), permanent magnet synchronous generators (PMSG) and others [2]. These technologies use power electronic converters and offer extensive controllability of both active and reactive power. They show less fluctuation in output power and can meet most grid code requirements. The disadvantage is their high costs [3]. The doubly fed induction generator (DFIG) is currently one of the most common wind turbine technologies installed in wind farm projects.
Recently, due to their technological advantages, the variable-speed wind turbines began to be widely used in new wind parks and became the dominant type used among installed wind turbines. However, the SCIGs are still used in many existing wind parks because of their lower price and higher operation reliability. Therefore, in the case of already installed wind parks, the immediate migration from one technology to another becomes economically impractical. The process of gradual modernization is more suitable.
Most papers study the operation characteristics of each wind park concept separately [4,5,6], and there is little research on the coordinated operation characteristics of wind parks containing both DFIGs and SCIGs. The authors in reference [7] investigate the coordinated operation of a wind park with different wind turbine concepts (fixed and variable speed) in order to guarantee the operational set points of active and reactive power specified by the Spanish transmission system operator. In [8], a coordinated control strategy is proposed for a wind park composed by DFIG and fixed speed induction generators to improve system stability during and after faults. Reference [9] analyzes the transient performance of a wind park with DFIG and SCIG with different rates of capacity; it considers DFIG operation with and without voltage control on rotor side converter. In [10], an investigation of the flicker emission is presented, considering a wind park with different types of wind turbines, fixed and variable speeds that operate under a variety of wind conditions and network characteristics. The results show the replacement of some fixed speed wind turbines with DFIG wind turbines can reduce the flicker emission in the system. Reference [11] investigates the control and operation of doubly fed induction generator and fixed-speed induction generator based wind farms under unbalanced grid conditions. The simulations are implemented in Matlab/Simulink.
Given what has already been investigated, we deem it necessary to undertake research that pays more attention to the operation characteristics of wind parks containing fixed speed turbines and variable speed turbines and how they affect the grid. In this context, this paper presents a detailed analysis of a mixed wind park containing both DFIGs and SCIGs. It follows a trend where an existing SCIG-based wind farm is being extended with DFIG turbines by the gradual replacement of some SCIG units by DFIG units. Different rates of capacity of DFIG and SCIG are considered, and the study addresses aspects of voltage stability, fault analysis, wind speed variation and critical fault clearance time. The simulations were conducted using the ANAREDE and ANATEM software that were developed by CEPEL [12,13]. The network used to perform the simulations is a real system in Brazil.
2. Models
This section presents the wind turbine models and induction generators that are used in this study. Two technologies are considered: squirrel cage induction generator and doubly fed induction generator. This section presents the turbines' technical details.
2.1. Wind turbine model
The wind turbine converts wind flow energy into mechanical energy. Depending on the wind speed, the turbine power output varies. The power extracted from a wind turbine (Pw) is proportional to the cube of the wind speed, and can be represented by the mathematical expression as follows:
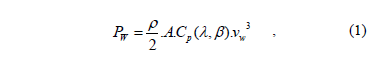
where r is the air density, A is the area swept by the rotor blades, vw is the wind speed, Cp is the power coefficient, b is the blade pitch angle, and l is the tip speed ratio [14]. Tip speed ratio can be expressed as the ratio between the speed of a blade tip (wT) and the wind speed (vw) as show in eq. (2), which considers the turbine radius R.

2.2. Squirrel Cage Induction Generator
Squirrel cage induction generators (SCIG) are fixed-speed wind turbines directly connected to the grid. They have a soft-starter and a capacitor bank to reduce reactive power compensation. Fig. 1 shows the general structure of a model of a constant-speed wind turbine. For power flow studies, the squirrel cage induction generator is modeled as a PQ bus. The induction generator model, which neglects the stator transients, is the same that is used in [15].
2.3. Doubly fed induction generator
Doubly fed induction generator (DFIG) is a variable-speed wind turbine that can achieve maximum aerodynamic efficiency over a wide range of wind speeds. In this configuration the stator is directly connected to the grid and the rotor is fed from a back-to-back AC/DC/AC converter set, as is shown in Fig. 2 [2]. The converter circuit allows the production or consumption of reactive power, making it different from the SCIG, which can only consume reactive power. Since the DFIG has reactive power controllability, it can operate on power factor control mode (PQ representation in load flow studies) or voltage control mode (PV representation in load flow studies). In this paper, voltage control mode was adopted, and DFIG was represented by a simplified third-order model in which electromagnetic transients of the stator are neglected [16].
The reactive power capability of DFIG changes with wind speed variation, and can be determined based on:

where QSmax is the stator reactive power limit, Ss is the stator apparent nominal power and Ps is the active power injected at the stator. A more detailed representation can be found in [5]. Then, during low wind speed conditions, which translate to lower values of active power, the reactive power injection limits are bigger. On the other hand, during high wind speed conditions, which translate to higher values of active power, the reactive power injection limits are lower.
3. Test system
The wind park used in this paper is shown in Fig. 3. This project is under construction in the city of Santana do Livramento, which is located in the Rio Grande do Sul state, Brazil [17]. This wind farm will be integrated into the Brazilian main system by two 230kV transmission lines that connect the cities of Alegrete and Santana do Livramento [18].
The wind system is compounded by DFIGs and SCIGs, which operate in parallel. The equivalent induction generators system has a 67MW capacity with 67 1 MW rated power wind turbines. The equivalent variable speed system has a 53.55MW capacity with 63 wind turbines based on a DFIG of 850kW rated power. All SCIGs were aggregated into a single equivalent system, and the incoming wind speed is considered to be identical on all the wind turbines. The DFIGs adopt the same equivalent method as that of SCIGs.
The loads are connected at substation Alegrete and Livramento, 20.0 MW/19.4 MVAr and 67.6 MW/19.3 MVAr respectively, and they are both modeled as constant power.
4. Simulation results
In order to analyze the behavior of mixed wind parks with different turbine types, four studies were conducted in this paper: voltage stability, fault analysis, wind speed variation and critical fault clearance time.
In most studies, five scenarios were analyzed to simulate the gradual modernization of the wind park, that is, the gradual replacement of SCIG wind turbines for DFIG wind turbines. The penetration level of wind generation SCIG and DFIG were modified simultaneously in order to maintain the active power -fixed at 50 MW- at the point of common coupling (PCC). The group of SCIG machines was connected to bus 9016 and the group of DFIG machines was connected to bus 9017. The initial wind speed was 10.14 m/s for both technology groups.
The results will now be presented, and the five scenarios considered were the following:
4.1. Voltage stability
Voltage stability margin (VSM) is the percentage difference between the system maximum loading point and the forecasted load, as is shown in eq. (4). It can be expressed in p.u., as a percentage, or in MW. This index is the most widely used indicator of system voltage security and is determined by using PV curve methods [19]. The PV curve can be obtained by using the continuation power flow method [20]. For each load increase, a load flow problem is solved, and the set of obtained equilibrium points defines the PV curve. The maximum loading point (critical point) corresponds to the system bifurcation point from the PV curve.
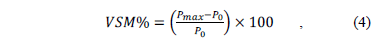
where P0 is the system initial load and Pmax is the maximum load.
Fig. 4 shows the PV curve for all scenarios analyzed. The results show that the system voltage stability margin increases as the DFIG penetration level also increases. This can be explained by the fact that voltage instability is mainly associated with reactive power support, and DFIG technology can supply reactive power to the system when operating in PV mode. It is important to mention that DFIG generators' maximum and minimum reactive power limits were modified for each scenario analyzed since the active power was also modified.
4.2. Fault analysis
A three-phase short-circuit fault was applied to 20% of the transmission line, which connects bus 9010 to bus 1230. The fault is applied at t = 2 seconds, and after 200 ms the fault is cleared. The simulation time is 10 seconds. In all simulations, the DFIG crowbar protection was activated.
Fig. 5 shows the results obtained by the SCIG group that was connected at bus 9016. During the fault, the terminal voltage drops very quickly. The voltage drop severity increases as the capacity of DFIG increases due to the reversal of reactive power flow. Moreover, the system recovery time is smaller due to DFIG reactive power support, which quickly reestablishes the electromagnetic torque. Also, as the capacity rate of DFIG increases, the reactive power absorbed by SCIG is smaller due to the DFIG's capacity to supply reactive power to the grid.
Fig. 6 shows the results obtained for the DFIG group that was connected at bus 9017. The fault also causes voltage sag, which is more severe due to the actuation of the crowbar protection scheme. This is responsible for opening the circuit between the rotor windings and rotor side converter, and interrupting the terminal voltage control. When the fault is cleared, the connection between the rotor side converter and the generator rotor circuit is restored, and terminal voltage control action is undertaken with immediate effect, which translated to the significant recovery of terminal voltage.
4.3. Wind speed variation
In all scenarios, the system was submitted to the same wind speed increase as is shown in Fig. 7: a positive step function of 2m/s applied at t = 2 seconds. The simulation time is 10 seconds. The crowbar protection of DFIG was not activated in all simulations. We considered the five scenarios previously presented with different capacity rates of DFIG and SCIG.
Fig. 8 shows the results obtained for the SCIG group that was connected at bus 9016. When the DFIG capacity rate increases and the SCIG decreases, the terminal voltage recovers more rapidly after the fault. Moreover, with the capacity rate of DFIG increasing, the recovery time and magnitudes of the oscillations are smaller. This is mainly because SCIG absorbs reactive power and DFIG can provide reactive power to the grid. As the simulation shows, the modernization of the wind park minimizes the undesirable effect of fixed speed wind turbines, which is typical oscillatory behavior. It is important to mention that all scenarios start with different initial conditions of reactive power consumption due to the progressive reduction on the number of SCIG generator units. Also, after the wind speed variation, the voltage does not return to its initial value as a result of SCIG capacitor banks.
Fig. 9 shows the results obtained for the DFIG group that was connected at bus 9017. The larger the DFIG rate of capacity, the more rapidly the voltage recovers after the fault. Also, in all scenarios, the voltage returned to its initial value due to DFIG capacity injecting reactive power that controlled terminal voltage and improved transient operation characteristics.
As wind speed increases, the active power produced by the wind generator also increases. Therefore, the DFIG reactive power limits decreases, as is shown in Fig. 10. It is important to mention that as DFIG penetration level increases, as expected, the reactive power available to provide reactive power support to the grid also increases. Also, wind park modernization provides an improvement in power system operation at the point of common coupling (PCC), as is shown in Fig. 11.
4.4. Critical fault clearance time
During severe faults, the electromagnetic torque of induction generators decreases when the mechanical torque related to wind speed is considered constant. In this case, the rotor shaft accelerated. When the fault is eliminated before the rotor speed reaches critical speed (when the electromagnetic torque corresponds to the same amount as before the disturbance), the rotor speed starts to decrease and the generator response is stable. Otherwise, when the fault is eliminated after the rotor speed reaches the critical speed, the rotor speed continues to increase and the generator will become unstable [21]. The time duration starting from the fault time until the critical slip point is the critical clearance time (CCT). The CCT is defined as the maximal fault duration for which the system remains transiently stable. The concept of the critical speed of induction generators was first proposed in [22] and further analyzed in [23]. During major faults in SCIG and DFIG, critical clearance time (CTT) is an important issue to consider.
The critical clearance time is conventionally evaluated using time-domain simulations, which involve several trials and repetitive runs of transient programs [24]. In this paper, the critical fault clearance time was calculated by gradually increasing the fault clearance time in steps of 5 ms. Two scenarios were then analyzed:
The first scenario considers that wind park is only based on SCIG technology. The second scenario considers that wind park is based on SCIG and DFIG technologies. The penetration level of wind generation SCIG and DFIG was fixed -at 50 MW- at the point of common coupling (PCC) for both scenarios. The group of SCIG machines, which remained installed in the wind park, was connected at bus 9016.
The results are presented in Table 1, and they reveal that the presence of DFIG technology in the wind park increases the critical clearance time, which is positive in terms of system stability.
Also, two simulations were performed in order to confirm the results. First, a network fault with the same duration of CCT (Tf = CCT) was considered. Then, a network fault with duration larger than CTT (Tf >> CCT) was assumed and the time-domain simulation was run once again. The results are presented in Fig. 12: they show scenario 1 (wind park composed by SCIG only) and scenario 2 (wind park composed by SCIG and DFIG). This figure reveals that, in the first scenario, when the short circuit is eliminated in or faster than 700ms, the wind generator does not lose its stability. Otherwise, when the fault clearance time is longer than 700ms, the wind generator becomes unstable. The same behavior is observed in scenario 2; this confirms that the critical fault clearance time was met.
5. Conclusions
This paper presents a behavioral analysis of a mixed wind park that is composed of doubly fed induction generators and squirrel cage induction generators. It considered different rates of capacity for each technology and simulated the gradual replacement of some SCIG units by DFIG units. The study addresses aspects of voltage stability, fault analysis, wind speed variation and critical fault clearance time. The network used to perform simulations is a real system in Brazil.
The results show that the parallel operation of DFIG and SCIG can improve voltage stability, increase fault clearance time, and improve the transient operation characteristics of the wind park and also the point of common coupling. According to the results obtained, gradual wind park modernization could be a cost-effective solution.
References
[1] Brasil Energy. A fast-growing market - Power special report. [Online]. February 2015. Available at: http://brasilenergy.editorabrasilenergia.com/.
[2] Jenkins N., Allan R., Crossley P., Kirschen D. and Strabac G., Embedded generation. The Institution of Electrical Engineers, London, United Kingdom, 2000. DOI: 10.1049/PBPO031E
[3] Alvarez J.M.G., Schwickardt G. and Targarona J.C.G., An overview of wind energy taking into consideration several important issues including an analysis of regulatory requirements for the connection of wind generation into the power system, DYNA, 79(172), pp. 108-117, 2012.
[4] Alvarez J.M.G. and Targarona J.C.G., Wind generation using different generators considering their impact on power system, DYNA, 78(169), pp. 95-104, 2011.
[5] Londero R.R., Affonso C.M. and Vieira J.P.A., Long-term voltage stability analysis of variable speed wind generators, IEEE Transactions on Power Systems, 30, pp. 439-447, 2015. DOI: 10.1109/TPWRS.2014.2322258
[6] Xu, L.Y.Z., Østergaard, J., Dong, Z.Y, Wong, K.P. and Ma, X., Oscillatory stability and eigenvalue sensitivity analysis of A DFIG wind turbine system. IEEE Transactions on Energy Conversion, 26, pp. 328-339, 2011. DOI: 10.1109/TEC.2010.2091130
[7] Rodríguez-Amenedo J.L., Arnaltes S. and Rodríguez M.A., Operation and coordinated control of fixed and variable speed wind farms. Renewable Energy, 33, pp. 406-414, 2008. DOI: 10.1016/j.renene.2007.03.003
[8] Foster, S., Xu, L. and Fox, B., Coordinated control and operation of DFIG and FSIG based wind farms, Proceedings of IEEE Power Tech, 2007, pp. 522-527. DOI: 10.1109/pct.2007.4538371
[9] Lin, L., Zhang, Y. and Yang, Y., Transient characteristics of the grid-connected wind power farm with DFIGs and SCIGs, Proceedings of Third International Conference on Electric Utility Deregulation and Restructuring and Power Technologies - DRPT, 2008, pp. 2676-2681. DOI: 10.1109/drpt.2008.4523864
[10] Gogdare, A.N., Doroudi, A. and Ghaseminejad, M., A new method to mitigate voltage fluctuation of a fixed speed wind farm using DFIG wind turbine. Proceedings of 17th Conference on Electrical Power Distribution Networks (EPDC), 2012, pp. 1-6.
[11] Wang, Y. and Xu, L., Coordinated control of DFIG and FSIG-based wind farms under unbalanced grid conditions. IEEE Transactions on Power Delivery, 25, pp. 367-377, 2010. DOI: 10.1109/TPWRD.2009.2033966
[12] CEPEL, Centro de Pesquisas de Energia Elétrica. ANAREDE: Programa de Análise de Redes. Manual Guide, 07-08 Version, August 1999.
[13] CEPEL, Centro de Pesquisas de Energia Elétrica. ANATEM: Análise de Transitórios Eletromecânicos. Manual Guide, 09 Version, December 2002.
[14] Slootweg, J.G., Polinder, H. and Kling, W.L., Dynamic modeling of a wind turbine with doubly fed induction generator. Proceedings of IEEE PES Summer Meeting, 2001, pp. 644-649.
[15] Rangel, D.R., Ferraz, J.C.R., Gomes Jr., S. e Henrique, R.M., Modelagem de geração eólica em programas de fluxo de potência e de análise de estabilidade eletromecânica. Proceedings of Seminário Nacional de Produção e Transmissão de Energia Elétrica - XVIII SNPTEE, 2005, pp. 1-8.
[16] Anaya-Lara, O., Jenkins, N., Ekanayake, J., Cartwright, P. and Hughes, M., Wind generation, modeling and control. John Wiley & Sons, Ltd., 2009.
[17] Soares, J., Jornal $$ - Parque eólico deve receber investimento de R$ 4,8 bi.. Porto Alegre, RS, [Online]. 2013. Available at: http://www.valor.com.br/brasil/3017264/parque-eolico-deve-receber-investimento-de-r-48-bi
[18] Pavan, A.P., Impacto Da geração eólica no desempenho dinâmico do sistema elétrico. MSc. Thesis, Universidade Federal de Santa Catarina, Florianópolis, SC, Brazil, 2006.
[19] Taylor, C., Power system voltage stability. New York: McGraw-Hill Inc., 1994.
[20] Kundur, P., Power system stability and control. New York: McGraw-Hill, 1994.
[21] Grilo, A.P., Mota, A.A., Mota, L.T.M. and Freitas, W., An analytical method for analysis of large-disturbance stability of induction generators. IEEE Transactions on Power Systems, 22, pp. 1861-1869, 2007. DOI: 10.1109/TPWRS.2007.907346
[22] Akhmatov, V., Knudsen, H., Bruntt, M., Nielsen, A.H., Pedersen, J.K. and Poulsen, N.K., A dynamic stability limit of grid-connected induction generators. Proceedings of International Conference on Power and Energy - IASTED, Spain, 2000, pp. 235-244.
[23] Akhmatov, V., Analysis of dynamic behavior of electric power systems with large amount of wind power. PhD. dissertation, Tech. Univ. Denmark, Lyngby, Denmark, 2003.
[24] Salman, S.K. and Teo A.L.J., Investigation into the estimation of the critical clearing time of a grid connected wind power based embedded generator, Proceedings of IEEE PES Transmission and Distribution Conference and Exhibition, 2002, pp. 975-980. DOI: 10.1109/TDC.2002.1177609
H.J.B. da Silva, received his BSc. in Technical Industrial from IFPA, Brazil, in 2011, and his MSc. degree from the Federal University of Para, Brazil, in 2014. At present, he is working towards a BSc. degree in Control and Automation Engineering at IFPA, Brazil. His research interests include wind power generation and power system stability. ORCID: 0000-0002-8260-1381
C. de M. Affonso, received her BSc. in Electrical Engineering from the Federal University of Para, Brazil, in 1998, her MSc. degree from the Federal University of Santa Catarina, Brazil, in 2000 and her PhD. degree in Electrical Engineering from State University of Campinas, Brazil, in 2004. Currently, she is a professor of electrical engineering at the Federal University of Para, Brazil. Her research interests are in power system planning and stability and distributed generation. ORCID: 0000-0002-2241-1711