
Felipe Mejía-Peláez* & Ignacio Vélez-Pareja**
* Internexa S. A. E.S.P. Medellín, Colombia E-mail: felipemejiap@gmail.com
** Universidad Tecnológica de Bolívar Cartagena, Colombia E-mail: ivelez@unitecnologica.edu.co
Submitted: September 2010 Accepted: July 2011
Abstract :
In this paper we propose an analytical solution to the circularity problem between value and cost of capital. Our solution is derived starting from a central principle of finance that relates value today to value, cash flow, and the discount rate for next period. We present a general formulation without circularity for the equity value (E), cost of levered equity (Ke), levered firm value (V), and the weighted average cost of capital (WACC). We furthermore compare the results obtained from these formulas with the results of the application of the Adjusted Present Value approach (no circularity) and the iterative solution of circularity based upon the iteration feature of a spreadsheet, concluding that all methods yield exactly the same answer. The advantage of this solution is that it avoids problems such as using manual methods (i.e., the popular "Rolling WACC") ignoring the circularity issue, setting a target leverage (usually constant) with the inconsistencies that result from it, the wrong use of book values, or attributing the discrepancies in values to rounding errors.
Keywords:
Firm valuation, cost of capital, cash flows, free cash flow, capital cash flow, WACC, circularity.
Resumen:
En este artículo proponemos una solución analítica al problema de la circularidad entre el valor y el costo de capital. Nuestra solución se obtiene a partir de un principio central de las finanzas que establece una relación entre el valor actual y el valor, el flujo de caja y la tasa de descuento en el siguiente período. Derivamos una formulación general del valor del patrimonio, P, del costo del patrimonio con deuda, del valor total y del costo promedio ponderado del capital, WACC, sin circularidad. Además, comparamos los resultados obtenidos usando estas fórmulas con los que resultan al usar el método de Valor Presente Ajustado, VPA (APV en inglés) sin circularidad, y la solución iterativa de circularidad basada en la función de iteración de una hoja de cálculo. Concluimos que todos los métodos producen el mismo resultado. La ventaja de esta solución es que evita problemas como el uso de métodos manuales (es decir, el popular "Rolling WACC") haciendo caso omiso de la cuestión de la circularidad, el establecimiento de un apalancamiento objetivo (target leverage) por lo general constante, con las inconsistencias que se derivan de ello, el uso inapropiado del valor en libros, o atribuir las diferencias entre los métodos de valoración a errores de redondeo.
Palabras clave:
valoración de empresas, costo de capital, flujos de caja, flujo de caja libre, flujo de caja de capital, WACC, circularidad.
Résumé :
Cet article propose une solution analytique du problème de la circularité entre la valeur et le coût de capital. La solution est obtenue à partir d'un principe central des finances établissant une relation entre la valeur actuelle et la valeur, le flux de caisse et le taux de décompte dans la période suivante. Nous dérivons une formulation générale de la valeur du patrimoine, P, du coût du patrimoine avec la dette, de la valeur totale et du coût pondéré moyen du capital, WACC sans circularité. De plus, les résultats obtenus utilisant ces formules sont comparés avec les résultats de l'utilisation de la méthode de Valeur Présente Ajustée, VPA (APV en anglais sans circularité), et la solution itérative de circularité basée sur la fonction d'itération d'une feuille de calcul. En conclusion, toutes les méthodes donnent le même résultat. Cette solution a pour avantage d'éviter des problèmes tels que l'utilisation de méthodes manuelles (le «Rolling WACC» populaire) tout en omettant la question de la circularité, l'établissement d'un levier objectif (target leverage) généralement constant, avec les inconsistances qui en dérivent, l'utilisation inappropriée de la valeur en livres, ou l'attribution des différences entre les méthodes de valorisation à des erreurs d'arrondissement.
Mots-clefs :
valorisation d'entreprises, coût de capital, flux de caisse, flux de caisse libre, flux de caisse de capital, wacc, circularité.
Resumo:
Neste artigo propomos uma solução analítica ao problema da circularidade entre o valor e o custo de capital. Nossa solução obtém-se a partir de um princípio central das finanças que estabelece uma relação entre o valor atual e o valor, o fluxo de caixa e a taxa de desconto no período seguinte. Derivamos uma formulação geral do valor do patrimônio, P, do custo do patrimônio com dívida, do valor total e do custo médio considerado do capital, WACC sem circularidade. Além disso, comparamos os resultados obtidos usando estas fórmulas com os que resultam da utilização do método de Valor Presente Ajustado, VPA (APV em inglês sem circularidade), e a solução iterativa de circularidade baseada na função de iteração de uma folha de cálculo. Concluímos que todos os métodos produzem o mesmo resultado. A vantagem desta solução é que evita problemas como o uso de métodos manuais (ou seja, o popular "Rolling WACC") ignorando a questão da circularidade, o estabelecimento de uma alavancagem objetiva (target leverage) geralmente constante, com as inconsistências daí derivadas, o uso inapropriado do valor em livros, ou atribuir as diferenças entre os métodos de valoração a erros de arredondamento.
Palavras Chave:
valoração de empresas, custo de capital, fluxos de caixa, fluxo de caixa livre, fluxo de caixa de capital, wacc, circularidade.
Since the Modigliani and Miller (1958) seminal paper, a problem has been identified related to the fact that the discount rate used to value cash flows depends on the value of the cash flows themselves. This gives rise to the Circularity Problem.
This problem has been addressed in different ways: Ignoring it and assuming a constant cost of capital, assuming that taxes do not exist and discounting the cash flows with the cost of capital before taxes, iterating manually assuming a target leverage, or iterating automatically using the iteration feature of spreadsheets.
In this paper we propose an analytical solution to this Circularity Problem. Our solution is derived starting from a basic tenet of finance as follows:

where V is value, CF is cash flow and DR is discount rate.
We derive a general formulation for the equity value (E) at a given period that depends on the value of debt (D) for the same period, and the values at the next period of equity and cash flow to equity (CFE), tax savings (TS) and its corresponding discount rate (ψ), the cost of debt (Kd), and the unlevered cost of equity (Ku). We then present this formula for two special cases: One for ψ equal to Kd, and another for ψ equal to Ku. In addition, we also derive formulations without circularity for the levered cost of equity (Ke), firm levered value (V), and weighted average cost of capital (WACC).
Authors, practitioners and teachers recognize the existence of the Circularity Problem and their proposed solutions range from iterative processes either manual ("Rolling WACC") or automated (using a spreadsheet), to using a target leverage or assuming constant WACC[1]. Other authors such as Benninga (2006) and Benninga and Sarig (1997) simply ignore the Circularity Problem and just use a constant WACC or Ke (Ku given that the tax shields are not considered), under the assumption that personal taxes approximately offset the tax shields from corporate taxes, which is in line with the findings reported by Miller (1977). Fama and French (1998) suggest a more challenging finding: Tax shields are not only negligible nor zero, but also exists a negative relation between leverage and total value.
Authors such as Lerner and Carleton (1966), Baginski and Wahlen (2003), Pfeiffer (2004), Rao and Stevens (2007), Vishwanath (2007), Apreda (2008), Woolley (2009), and some practitioners, recognize the existence of circularity but do not offer a solution to the problem. Rao and Stevens recognize the existence of such circularity and state that "prior research has noted, but not modeled these interactions". (Rao and Stevens, 2007, p. 2).
Vishwanath (2007) recognizes that using book value and market values when introducing the leverage in the WACC yield different results; he asserts that "The market value of equity is the present value of equity cash flows but the discount rate used to discount ECFs itself is supposed to be based on the market value of equity. That is, there is a circularity problem. We can get over this problem by using the quasi market valuation". (Vishwanath, 2007, p. 559). This solution, which accepts different results, is not adequate (as neither are many others), not because the valuation process is exact (which is not), but because the differences in the results obtained by using different methods leave the analyst with the uncertainty of whether the results differ in fact due to intrinsic properties of the methods themselves or to faults in their application (see Vélez- Pareja, 2006, for an example of the magnitude of the discrepancies using constant WACC and ignoring the effect of changes in leverage on WACC).
As noted above, even practitioners acknowledge the Circularity Problem: "Now, to be able to calculate WACC we need to know the value of the company, but to calculate that value we need to know WACC. So we have a circularity problem involving the simultaneous solution of WACC and company value". (Strategy @ Risk, Visited March 19, 2010). However, as shown below, there are solutions to this problem.
There are many authors who propose a target capital structure and/or an iterative solution departing from an initial target leverage[2]. According to Crundwell (2008), "The values for debt and equity used in calculation of the WACC must be market values (not historical values) and they must be targeted values [...] not current values. This circular argument creates difficulties" (Crundwell, 2008, p. 378). Koller et al. (2005) are straightforward: "To value the company, use target weights;" however, at the same time, they argue that "you must determine equity value (for the cost of capital) either using a multiples approach or through DCF iteratively. To perform an iterative valuation, assume a reasonable capital structure, and value the enterprise using DCF. Using the estimate of debt to enterprise value, repeat the valuation. Continue this process until the valuation no longer materially changes". (Koller et al., 2005, pp. 324-325). This proposal lacks logical consistency: If the multiples approach is an acceptable and equivalent procedure, the analyst does not need to perform any additional procedure. On the other hand, if the two methods are not equivalent, the analyst must choose the "proper" procedure.
Pinteris (n. d.) states: "Note that the choice of a target capital structure is also dictated by the presence of a circularity problem in our calculations. In order to estimate the WACC we need to know the market weights of debt and equity. In order to do so, we need to know, in particular, the market value of equity. But this depends on the discount rate used to discount future free cash flows, which is given by the WACC. Estimating the target capital structure, we could use the current market-based capital structure of the company and review the capital structure of similar companies, as well as examine the management's policy towards financing". (Pinteris, n. d., p. 5). Berk and Demarzo (2009) recognize that when leverage changes, the WACC changes and the value is difficult to calculate; in order to solve this they calculate the value with the APV and then compute the WACC. They then use this WACC to calculate value with the FCF and obviously obtain the same value. Since APV is the easiest way to solve circularity, why is it necessary to calculate WACC after calculating value with the APV? Although it is not exactly the case, we recognize that the application of the formula for WACC using the results of a first method breaks the circularity, but it does not make sense: If the purpose is to value the firm and the analyst already knows it when using APV, it should not be necessary to repeat the process using the value already known to obtain the same value using the FCF and the WACC. When using discounted cash flow methods, all methods should be consistent and self-contained.
In an introduction to his defense of APV, Luehrman says: "One expedient is to guess at the market value or use book values and then iterate -fill in the computer market value as the new guess, then recompute another guess, and so forth until the guess and the computed values converge". (Luehrman, 1997, p. 153). Professor Abarbanell (1999, p. 6) warns the reader: "[...] plugging the actual market value of the firm into the calculation of WACC involves circular reasoning (since we are trying to determine what that market value should be!). Thus, it is necessary to guess at the firm's market value, use the guess to determine the weights to apply in the WACC, and determine if the estimated WACC leads to a projected equity value of the firm equal to your original guess". This is also known as "Rolling WACC".
Damodaran (2000) recognizes that "every textbook is categorical that the weights in the cost of capital calculation be market value weights" and that the problem is the "inconsistency" behind this. To solve this inconsistency he proposes an iterative procedure. This is the "Rolling WACC" that eventually "will converge sooner rather than later".
Copeland et al. (2000, p. 204) consider that "the second reason for using a target capital structure is that it solves the problem of circularity involved in estimating the WACC". Nevertheless, this is a simplistic argument: Target leverage cannot go in one way while actual leverage goes another, which happens when using target leverage and not adjusting debt according to it. Brealey and Myers (2003, pp. 227 and 25) avoid the issue of circularity assuming that they have a balance sheet with market values. If that is the situation, then they do not need to calculate WACC. They even mention an industry cost of capital (Brealey and Myers, 2003, p. 550), but this does not solve the problem either, and is an incomplete approach having that cost of capital is affected by firm-specific variables that might differ from the industry as a whole.
Wood and Leitch (2004) state that "There is no general analytical solution to this circularity, so the ordinary weighted average cost of capital cannot capture the effects of changing capital structure on the cost of capital, and the computed NPV is not correct: The wealth of the shareholders will change by a different amount, and may have a different sign as well". (Wood and Leitch, 2004, p. 16). They also affirm that "Such circularity precludes a general analytical solution to the problem of determining the appropriate discount rate to use for a proposed project. The FPV solution technique uses an iterative method to attack this circularity". (Wood and Leitch, 2004, p. 19). This paper is a direct answer to these asserts by Wood and Leitch (2004). Also, Vélez-Pareja and Tham (2005) published a reply to that paper.
Pratt (2002) comments that "in computing WACC for a closely held company, project, or proposed project, one important additional problem exists: Because there is no market for the securities, we have to estimate market values in order to compute the capital structure weightings. As we will see, estimating the weightings for each component of the capital structure becomes an iterative process for companies intending or assumed to operate with current levels of debt. Fortunately, computers perform this exercise very quickly. To 'iterate' means to repeat. An 'iterative process' is a repetitious one. In this case, we estimate market value weights because the actual market values are unknown. We may re-estimate weights several times until the computed market value weights come fairly close to the weights used in estimating the WACC". (Pratt, 2002, pp. 48-49). Again, this is the "Rolling WACC" referred to above.
After recognizing the existence of circularity, Abrams (2001, p. 180) mentions that "using an iterative approach eliminates this deficiency in both models. After determining the market value of debt, we can assume any value for equity to get our initial debt to equity ratio. We calculate the first iteration of equity value using this initial ratio. After several iterations, we eventually obtain a unique solution for equity that is consistent with the last iteration of the debt to equity ratio and is independent of our initial choice of equity", being this another example of Rolling WACC. Finally, Damodaran (n. d.) in one of his teaching slides recommends:
"Rather than use book value weights, you should try
As shown, the Rolling WACC approach has many revered advocates.
According to Lazar and Prisman (2006), "This introduces circularity into the process as if the market value of the debt and equity are known so is the value of the firm, but the value of the firm is what we try to estimate. Even in valuing a firm practitioners use book values as a solution to this problem even though it can be solved numerically. A few iterations can obtain a value of equity and debt that is consistent (to a tolerance) with the value of the firm". (Lazar and Prisman, 2006, p. 24). Greenwald -when commenting on appraising regulatory projects- mentions that "the basic difficulty in valuing a utility's assets (i.e., its rate base) is one of circularity. Their value is determined, like those of any asset, by the net income they are capable of producing. But, this in turn is determined by the policies of the relevant regulatory agency and, in particular, by the value such an agency places on the assets of the utility. Thus, valuations by a regulatory authority tend to be selffulfilling and there is no firmly based principle by means of which this circle can be broken. Attempts to break it have traditionally taken two directions". (Greenwald, 1980, p. 2). A similar problem was posed and solved by Vélez-Pareja (2006). With the computing facilities we have today this is a simple problem that can be solved using what they call reverse engineering: Set the Net Present Value to zero changing the tariffs of the given utility.
Vélez-Pareja and Tham (2009), Tham and Vélez-Pareja (2004), and Vélez-Pareja and Burbano-Pérez (2010) have proposed the solution to circularity constructing the circular relation and iterating using the spreadsheet ability to handle such iterative process.
Reporting the results of a survey on tools used in capital budgeting, Truong et al. (2008, pp. 107 and 118) explain that "most respondents (84%) estimated a WACC. In computing the WACC, 60% of companies said they used target weights and 40% used current weights. In regard to the choice between market value and book value weights there was a substantial drop in the number of respondents. Those companies that responded show a nearly even balance between those who used market value weights (51%), and those who used book value weights (49%)". On the other hand, "the project cash flows are discounted at the weighted average cost of capital as computed by the company, and most companies use the same discount rate across divisions. The discount rate is assumed constant for the life of the project. The WACC is based on target weights for debt and equity".
Others use or modify a simple solution proposed by Myers (1974), the Adjusted Present Value, APV. For instance, Luehrman (1997) advocates for Myers' APV; McDaniel (1994, p. 147) considers that "the APV method of dealing with flotation costs by adjusting the initial investment is feasible for a general capital budgeting/financing case, because circularity can be avoided by using an algorithm that matches each project's NPV with the incremental flotation cost of the security potentially issued to finance the project. The APV method reduces the ambiguity of the stock price variable in the Gordon model. However, without modification, the APV method may reject value-increasing strategies for those firms with promising long-range investment opportunities". On the other hand, Adserà and Viñolas (2003) recognize the existence of circularity for perpetuities and propose a modified version of APV as the solution. It is clear that solutions like APV by Myers, (1974), and Capital Cash Flow, CCF, proposed by Ruback (2002), are good solutions under some conditions regarding the discount rate for the tax shields as will be seen below. In the case of APV, once the analyst has defined the discount rate for the tax shield, the procedure is straightforward. This is also the case of CCF, which requires a simple calculation when the discount rate for TS is Ku, the cost of unlevered equity.
In consequence, the previously cited literature clearly depicts how many practitioners and academics use the WACC. Finally, Vélez-Pareja and Benavides (2006) present an analytical solution to the circularity that derives into the Capital Cash Flow.
The idea of using target leverage is to elude or avoid the circularity problem, or if accompanied by an iteration process, to solve it. Those who elude the problem with the straightforward use of target leverage without any iteration presume they are correctly avoiding the problem. In fact, when we assume a target leverage, usually considered constant, we have circularity because the general formulation of cost of capital (be it the levered equity cost of capital, Ke, or the weighted average cost of capital, WACC) depends on the tax savings and/or their market value. Hence, we need to calculate debt in period "t-1" for the cost of capital in "t" and from there until the end of the planning horizon. The current practice dismisses this situation and applies the standard textbook formula, ignoring the fact that it should not be done without the rebalancing of debt and the resulting effect on the value of tax savings (see Tham and Vélez-Pareja, 2004, and Taggart, 1991).
It should be noted that if the rebalancing of debt is not undertaken, the cash flow to equity, CFE, cannot be calculated (assuming correctly that CFE is what the shareholder effectively receives) (See Magni and Vélez-Pareja, 2009).
Using the basic tenet of finance and the derivation for the levered cost of equity by Taggart (1991) and Tham and Vélez-Pareja (2004), we analytically solve the problem of circularity between the capital structure and the required rate of return. We assume that debt schedule is known from the beginning and could have any kind of profile. A "known" debt schedule is the result of solving the needs of cash when short- and long-term deficits are modeled in a financial planning model (see Vélez-Pareja, 2009). These formulae are derived in Appendix A.
A general formula for any discount rate for TS, ψ is
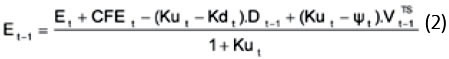
and

where E is the market value of equity, CFE is the cash flow to equity, Kd is the cost of debt, Ku is the unlevered cost of equity, D is market value of debt, ψ is the discount rate of the tax savings, TS, V is the market value of the firm, VTS is the market value of TS, FCF is free cash flow, and the subindices "t-1" and "t" denote two consecutive periods.
For the special case ψ = Kd we have
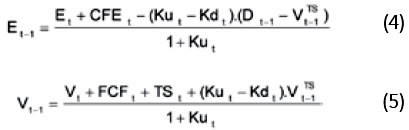
And for ψ = Ku,
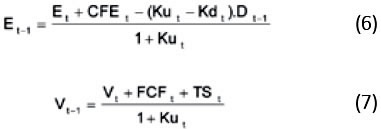
Observe that equation (7) is the value calculated with the capital cash flow, CCF, proposed by Ruback (2002), being a basic tenet of finance, as mentioned in equation (1).
The general formula for WACC is

For ψ = Kd we have

And for ψ = Ku,

The expression without circularity for Ke is

For ψ = Kd
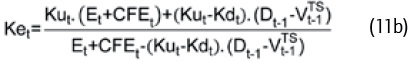
For ψ = Ku

Tham and Vélez-Pareja (2004) propose a calculation for the terminal value that solves circularity. The formulation is Terminal value for tax savings, VTV _TS in N is

where T is corporate tax rate, Kd is cost of debt, D%N is leverage at the end of the forecasting period, Ku is unlevered cost of equity and g is nominal growth (all of these variables are at perpetuity) and VTV_LN is levered firm terminal value.
In this case we assume that for perpetuity, Earnings before Interest and Taxes, EBIT, are greater than financial expenses, that taxes are paid the same year as accrued, and that interest is the only source of tax savings (see Vélez-Pareja, 2010). When these conditions are met we can say that tax savings are equal to TKdDt-1.
The formula for the unlevered TV is
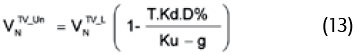
Solving for the levered terminal value we have

where FCFN+1 is the free cash flow at N+1 and φ is

With this collection of formulae we solve analytically the circularity problem.
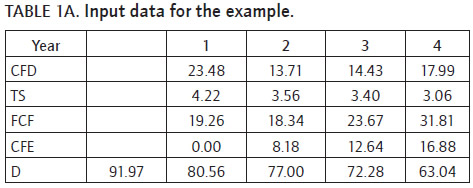
In this example we assume ψ = Ku. In Appendix B we repeat this example for ψ = Kd. In Table 1A we present the input data.
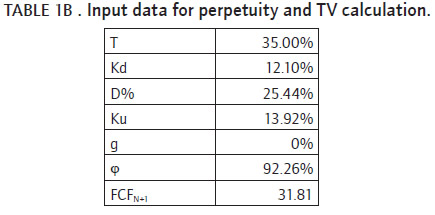
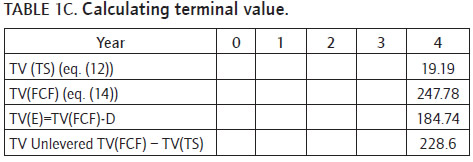
Terminal Value for the firm and for the TS was calculated using equations (12) and (14) and input data from Table 1B. We show the results in Table 1C.
In Table 2 we calculate the market value of equity using equation (6):


In Table 3 we calculate firm value using equation (7) and from it, we compute market value of equity:


As expected, the two values are identical.

In Table 4 we calculate firm value using FCF and WACC from equation (10):

Again, as expected, firm and equity values are identical to the ones found in previous approaches.
Now, using (11c) we calculate the market value of equity using CFE and Ke without circularity in Table 5.
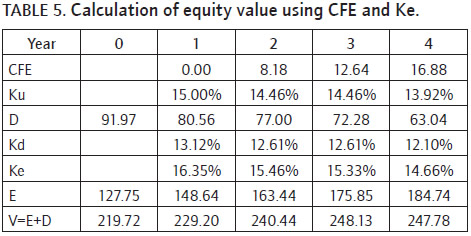
Once more, as expected, market values match.
As APV is the simplest way to calculate value without circularity, we check our results with the APV and ψ = Ku in Table 6, which shows that the proposed analytical method gives consistent results with the former.

In addition, we have tested the formulas with three valuations of real cases. One of them (a telecommunications project) presents typical problems that include complexities such as unpaid taxes, losses carried forward, foreign exchange debt, presumptive income, and inflation adjustments to the Financial Statements (see Vélez-Pareja and Tham, 2010).The other two (a construction firm and a restaurant) involve losses carried forward.
Regarding the first case, it is relevant to mention that when there are sources of TS different from interest expenses and periods where there is no debt, one could expect that WACC is the unlevered cost of equity, Ku; however this is not true due to the fact that the general expression for WACC has the value of TS (VTS) involved in it, regardless of their source. The other two cases are similar with the exception that there are no TS from different sources. Hence, when there is no debt, WACC is equal to Ku.
In all cases it is important to keep in mind that when dealing with TS and VTS in the formulas, any TS has to be included in the formulation, when they occur. This is particularly true when there are losses carried forward that allow "lost" TS to be recovered in future periods.
Moreover, the use of traditional textbook formula for WACC presents problems given that it is valid for a very restricted case and this causes many inconsistencies. These inconsistencies have to be solved defining an "effective" corporate tax rate that takes into account the effect of the above mentioned complexities.
We have shown four analytical solutions for the circularity problem, namely, the calculation of equity market value and the total firm value without the need of computing the values WACC or Ke, the computation of firm value using WACC without circularity along with FCF, and market value of equity without circularity by means of Ke and CFE. We have also shown that the solution (valuation) using the proposed methods is consistent, given an assumption on the discount rate to be used for the TS. All the methods coincide with the APV, which is the best method to calculate value without circularity. These methods do not require neither target leverage nor iterations.
We have mentioned some advantages of using the proposed solution of circularity. We stress that this solution solves the conundrum-type procedures that we have witnessed during decades. Although the herein proposed methods should yield identical results, it is advisable to test any solution (as we have done the presented examples) with simple procedures such as APV and CCF.
Practitioners have preferences regarding valuation methods. For instance, they might lack confidence in dealing with CFE discounted with Ke, instead of working with the venerable FCF and WACC. Others do not trust the APV or consider that this method is only suitable for certain type of situations. However, they may all profit from a direct and straightforward procedure that yields the correct answer using a spreadsheet.
Finally, skeptical readers or practitioners might perceive this procedure as non-intuitive and cumbersome. Nevertheless, management should abandon the position of finding simple or "magical" three-letter solutions to complex problems, which, by their very nature, do not have straightforward answers. Valuation and the use of valuation models should be seen as tools to actually make value based management yield tangible results.
[1] Constant leverage does not grant constant levered cost of equity (Ke) and WACC as both depend on the value of TS (Vélez-Pareja et al., 2008).
[2] Rosenberg and Guy (1976), Greenwald (1980), Luehrman (1997) (as an introduction to his defense of the Adjusted Present Value, APV), Abarbanell (1999), Copeland et al. (2000), Abrams (2001), Pratt (2002), Brealey and Myers (2003), Hitchner (2003), Schiefner and Schmidt (2003), Schuster and Jameson (2003), Froidevaux (2004), Schultze (2004), Tham and Vélez-Pareja (2004), Wood and Leitch (2004), Mello-e-Souza and Bee (2005), Hua and Upneja (2005), Koller et al. (2005), Damodaran (n. d., slides, 2000, 2006), De- Mario and Fazzone (2006), Lazar and Prisman (2006), Vélez-Pareja (2006), Mohanty (2007), Penman (2007) (cited by Liu, 2009), Crundwell (2008), Mian and Vélez-Pareja (2008), Pratt (2008), Pratt and Grabowski (2008), Turner (2008), Ansay (2009), Berk and Demarzo (2009), Hess et al. (2009), Liu (2009), Lobe (2009), Vélez- Pareja and Tham (2005, 2009), Vélez-Pareja and Burbano-Pérez (2010), Fairchild (n. d.), Pinteris (n. d.), Mathiesen (n. d.), Tijdhof (n. d.), and the Center for Financial Research.
Fundamental and independent equations:
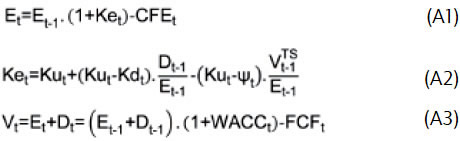
From Taggart (1991) and Tham and Vélez-Pareja (2004),
we have the general formula for WACC:

General expression for E for any ψ:
From equation (A1) we have

Replacing equation (A2) into equation (A5)
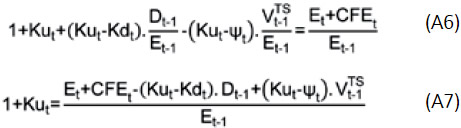
Simplifying and comparing with equation (A1), we solve
for E, the market value of equity:
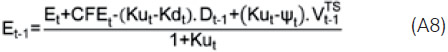
Equity value when ψ=Ku:

Equity value when ψ=Kd:
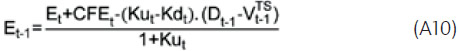
Now we find the Cost of Equity. Replacing equation (A8)
in equation (A5) we have:

Simplifying, we have the formula for the Cost of Equity Ke:

Cost of Equity when ψ=Ku:

Derivation for WACC and V = E + D
General expression for WACC for any ψ:
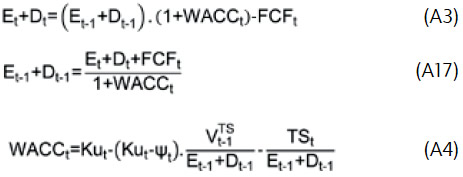
Replacing (A17) in (A4):
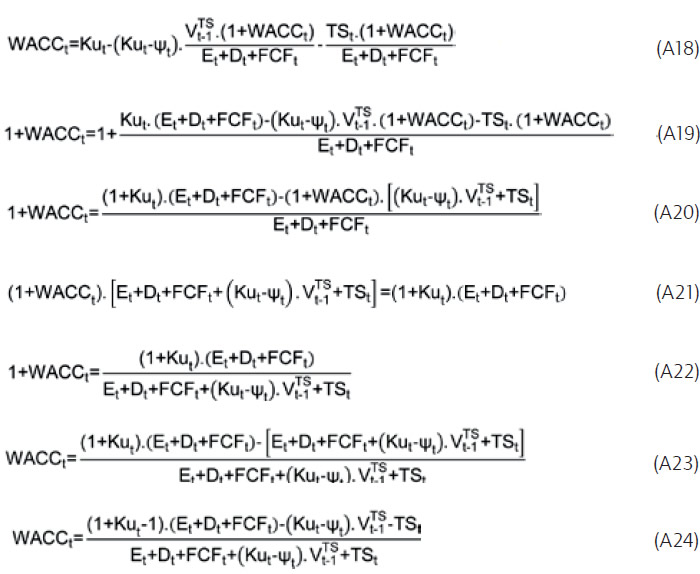
Simplifying, we have the formula for the WACC without circularity:

WACC when ψ=Ku:

General expression for V = D + E for any ψ:
Replacing equations (A22) in (A17):

Simplifying, we now have the formula for V = E + D without circularity:
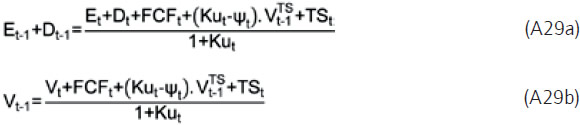
Formula for V = E + D when ψ=Ku:

Since FCFt + TS t = CFEt + CFDt, where CFDt is the cash flow
to the debt holders, this is the basic tenet of finance applied
to the Capital Cash Flow.
Formula for V = E + D when ψ=Kd:

Example assuming ψ = Kd. In Table B1 we present the input
data for the example.

In Table B2 we calculate market equity value directly with
equation (A10).
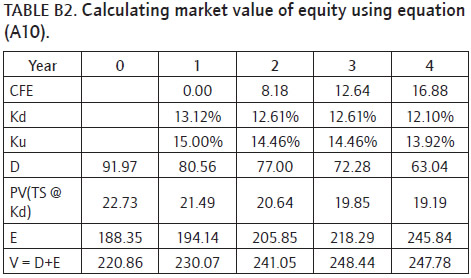
As expected, the two values (for firm and equity) are identical.
Table B3 shows the calculation of firm value directly using
equation (A31).
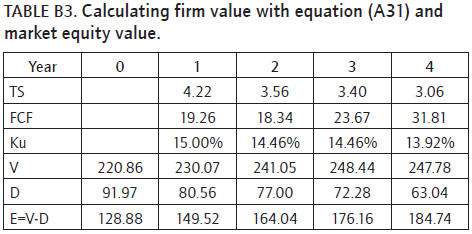
Using equation (A27) and the FCF we calculate firm value
and equity value in Table B4.

Again, all values match.
Now in Table B5 and using equation (11c) we calculate
equity market values from CFE and Ke without circularity.

Once more, as expected, market values match.
As APV is the simplest way to calculate values without circularity,
we show its calculations in Table B6. As expected,
all previous calculations coincide with APV.

Abarbanell, J. (1999). Valuing the gap using a discounted cash flow model. Handout for the purpose of class discussion. Hass Graduate School, University of Berkeley. Retrieved from http://www.haas.berkeley.edu
Abrams, J. B. (2001). Quantitative business valuation: A mathematical approach for today's professional. New York: McGraw-Hill.
Adserà, X. & Viñolas, P. (2003). FEVA: A financial and economic approach to valuation. Financial Analysts Journal, 59(2), 80-87.
Ansay, T. (2009). Firm valuation: Tax shields and discount rates. Memoire Présenté en vue de l'obtention du Master en Ingénieur de gestion, à finalité spécialisée.
Apreda, R. (2008). Cost of capital adjusted for governance risk through a multiplicative model of expected returns. Working paper, downloadable from http://ideas.repec.org/p/cem/doctra/383.html.
Baginski, S. P. & Wahlen, J. M. (2003). Residual income risk, intrinsic values, and share prices. The Accounting Review, 78(1), 327-351.
Benninga, S. (2006). Principles of finance with excel. New York: Oxford.
Benninga, S. & Sarig, O. (1997). Corporate finance: A valuation approach. New York: McGraw-Hill.
Berk, J. B. & Demarzo, P. (2009). Corporate finance: The core. Boston, MA: Pearson.
Brealey, R. A. & Myers, S. C. (2003). Principles of corporate finance. New York: McGraw-Hill.
Copeland, T., Koller, T. & Murrin, J. (2000). Valuation. Measuring and managing the value of companies, 3rd ed. Hoboken, N.J.: John Wiley & Sons Inc.
Crundwell, F. K. (2008). Finance for engineers: Evaluation and funding of capital projects finance for engineers. London: Springer-Verlag London Limited.
Damodaran, A. (2000). The dark side of valuation. Hoboken, N.J.: John Wiley & Sons Inc. http://pages.stern.nyu.edu/~adamodar/pdfiles/papers/HighGrow.pdf
Damodaran, A. (2006). Valuation approaches and metrics: A survey of the theory and evidence. (pp. 30-31). http://pages.stern.nyu.edu/~adamodar/pdfiles/papers/valuesurvey.pdf.
Damodaran, A. (n. d.). Valuation. Slide 46. Available at www.stern.nyu.edu/~adamodar/pptfiles/eq/dcfvegN.ppt.
DeMario, M. & Fazzone, A. P. (2006). The adjusted present value: An alternative approach to the effect of debt of business value. Business Valuation Update, 12(12), Available at http://www.bvresources.com/BVWireCentral/Material/WO112706/1206BVUDeMarioFazzone.pdf.
Fairchild, K. (n. d.) The cost of capital & discount rates. Available at http://faculty.business.utsa.edu/kfairchild/classes/5023/Lectures/5023Lectures.htm.
Fama, E. & French, K. (1998). Taxes, financing decisions, and firm value. The Journal of Finance, 53(3), 819-843.
Froidevaux, P. (2004). Fundamental equity valuation: Stock selection based on discounted cash flow. Thesis presented to the Faculty of Economics and Social Sciences of the University of Fribourg (Switzerland) in fulfillment of the requirements for the degree of Doctor of Economics and Social Sciences, Accepted by the Faculty's Council on 1 July 2004.
Greenwald, B. C. (1980). Admissible Rate Bases, Fair Rates of Return and the Structure of Regulation. The Journal of Finance, 35(2), 359-368.
Hess, D., Homburg, C., Lorenz, M. & Sievers, S. (2009). Extended dividend, cash flow and residual income valuation models - Accounting for deviations from ideal conditions (April 9). Available at SSRN: http://ssrn.com/abstract=1145201.
Hitchner, J. R. (2003). Financial valuation: Applications and models. Hoboken, N.J.: John Wiley & Sons Inc.
Hua, N. & Upneja, A. (2005). True market value of lodging stocks: A convergence approach. Journal of Hospitality Financial Management, 13, 1. Available at http://scholarworks.umass.edu/jhfm/vol13/iss1/14/ Visited March 19, 2010.
Koller, T., Goedhart, M. & Wessels, D. (2005). Valuation: Measuring and managing the value of companies - McKinsey & Co. Hoboken, N.J.: John Wiley & Sons Inc.
Lazar, F. & Prisman, E. (2006). Calculating the cost of capital for LDCs in Ontario. Teaching slides available at http://www.oeb.gov.on.ca/documents/cases/EB-2006-0088/4coc_lazarprisman_210606.pdf
Lerner, E. M. & Carleton, W. T. (1966). Financing decisions of the firm. The Journal of Finance, 21(2), 202-214.
Liu, Y. C. (2009). The slicing approach to valuing tax shields. Journal of Banking & Finance, 33, 1069-1078.
Lobe, S. (2009). Caveat WACC: Pitfalls in the use of the weighted average cost of capital. Corporate Ownership and Control, 6(3), 45-52.
Luehrman, T. (1997). Using APV: A better tool for valuating operations. Harvard Business Review, (May-June), 145-154.
Magni, C. A. & Vélez-Pareja, I. (2009). Potential dividends versus actual cash flows in firm valuation. The ICFAI Journal of Applied Finance, 15(7), 51-66. Available at SSRN http://papers.ssrn.com/abstract=1374070.
Mathiesen, H. (2010). Presentation: Fundamental value analysis. Available at http://e.viaminvest.com/A2MonitorSystems/AppA2MonitorSystems/Pres_FundamentalValueAnalysis.asp.
McDaniel, W. R. (1994). Techniques for including flotation costs in capital budgeting: Materiality, generality and circularity. Financial Practice and Education, (Spring/Summer), 139-148.
Mello-e-Souza, C. A. & Bee, S. (2005). Business valuation software: A blueprint for reliability (November 6). Available at SSRN: http://ssrn.com/abstract=844543
Mian, M. A. & Vélez-Pareja, I. (2008). Applicability of the classic WACC concept in practice. Latin American Business Review, 8(2), 19-40, Available at SSRN: http://ssrn.com/abstract=804764.
Miller, M. H. (1977). Debt and taxes. Journal of Finance, 32(2), 261-276.
Modigliani, F. & Miller, M. H. (1958). The cost of capital, corporation taxes and the theory of investment. The American Economic Review, XLVIII, 261-297.
Mohanty, P. (2007). Solving the circularity problem in estimating the cost of capital: A practical approach. The Icfai Journal of Applied Finance, 13(2), 29-38.
Myers, S. C. (1974). Interactions of corporate financing and investment decisions-implications for capital budgeting. The Journal of Finance, 29(1), 1-25.
Penman, S. H. (2007). Financial statement analysis and security valuation, 3rd ed. New York: McGraw-Hill.
Pfeiffer, T. (2004). Net present value-consistent investment criteria based on accruals: A generalisation of the residual income-identity. Journal of Business Finance & Accounting, (September/October), 910-911.
Pinteris, G. (n. d.). Notes on weighted-average cost of capital (WACC). Finance, 422, Department of Finance College of Business University of Illinois at Urbana-Champaign. Available at http://www.business.uiuc.edu/gpinteri/wacc.pdf.
Pratt, S. P. (2002). Cost of capital: Estimation and applications, 2nd ed. Hoboken, New York: John Wiley & Sons Inc.
Pratt, S. P. (2008). Valuing a business: The analysis and appraisal of closely held companies, 5th Ed. New York: McGraw-Hill.
Pratt, S. & Grabowski, R. (2008). Cost of capital: Applications and examples, 3rd Edition. Hoboken, N.J.: John Wiley & Sons Inc.
Rao, R. K. S. & Stevens, E. C. (2007). A theory of the firm's cost of capital: How debt affects the firm's risk, value, tax rate, and the government tax's claim. Singapore: World Scientific Publishing Co. Pte. Ltd.
Rosenberg, B. & Guy, J. (1976). Prediction of beta from investment fundamentals. Part One, Prediction criteria. Financial Analysts Journal, 32(3), 60-72.
Ruback, R. S. (2002). Capital cash flows: A simple approach to valuing risky cash flows. Financial Management, 31(2) (Summer), 85-103.
Schiefner, L. & Schmidt, R. (2003). Shareholder value at risk: Concept for company valuation, implementation, and simulation example. Available at http://www2.wiwi.uni-halle.de/wiwi/lui/bwl/bank/schmidt/MLU-WIWI-WP47-2003.pdf
Schultze, W. (2004). Valuation, tax shields and the cost of capital with personal taxes: A framework for incorporating taxes. International Journal of Theoretical and Applied Finance, 7(6), 769-804.
Schuster, P. & Jameson, M. (2003). The past performance and future value of companies. Management Accounting Quarterly, 4(4). Available at http://www.imanet.org/pdf/1836.pdf.
Strategy @ Risk, The weighted average cost of capital. Available at http://www.strategy-at-risk.com/2008/09/08/the-weightedaverage-cost-of-capital/.
Taggart, Jr., R. A. (1991). Consistent valuation cost of capital expressions with corporate and personal taxes. Financial Management, (Autumn), 8-20.
Tham, J. & Vélez-Pareja, I. (2004). Principles of cash flow valuation. An integrated market-based approach. Boston: Academic Press.
Tijdhof, L. (n. d.) WACC: Practical guide for strategic decision-making - Part 1: Is estimating the WACC like interpreting a piece of art? http://www.zanders.nl/publicaties/documents/WACC_part1.pdf (Visited March 20, 2010)
Truong, G., Partington, G. & Peat, M. (2008). Cost-of-capital estimation and capital-budgeting practice in Australia. Australian Journal of Management, 33(I) (June), 95-121.
Turner, J. (2008). The circularity problem with free cash flow. Available at SSRN: http://ssrn.com/abstract=1095227.
Vélez-Pareja, I. (2006). Valuating cash flows in an inflationary environment. The case of World Bank. In B. T. Credan (ed.), Trends in Inflation research. (pp. 87-141). New York: Nova Publishers.
Vélez-Pareja, I. (2009). Constructing consistent financial planning models for valuation (August 15). IIMS Journal of Management of Science, 1(January-June 2010) (Inaugural Issue), 1-26. Available at SSRN: http://ssrn.com/abstract=1455304
Vélez-Pareja, I. (2010). Risky tax shields and risky debt: An exploratory study. Cuadernos de Administración, 23(41), 213-235.
Vélez-Pareja, I. & Benavides, J. (2006). There exists circularity between WACC and Value? Another solution. Estudios Gerenciales, 98(January-March), 13-23.
Vélez-Pareja, I. & Burbano-Pérez, A. (2010). Consistency in valuation: A practical guide. Academia, Revista Latinoamericana de Administración, 44(May), 21-43.
Vélez-Pareja, I. & Tham, J. (2005). Proper solution of circularity in the interactions of corporate financing and investment decisions: A reply to the financing present value approach. Management Research News, 28(10), 65-92.
Vélez-Pareja, I. & Tham, J. (2009). Market value calculation and the solution of circularity between value and the weighted average cost of capital WACC. RAM - Revista de Administração Mackenzie, 10(6). Retrieved from http://www3.mackenzie.br/editora/index.php/RAM/issue/view/38
Vélez-Pareja, I. & Tham, J. (2010). Company's valuation in an emerging economy - Case Study TIMAN CO S. A. The Valuation Journal, 5(2), 4-45.
Vélez-Pareja, I., Ibragimov, R. & Tham, J. (2008). Constant leverage and constant cost of capital: A common knowledge half-truth (June 29). Estudios Gerenciales, 24(107), 13-34. Available at SS RN: http://ssrn.com/abstract=997435.
Vishwanath, S. R. (2007). Corporate finance: Theory and practice, 2nd ed. New Delhi: Response Books.
Wood, J. S. & Leitch, G. (2004). Interactions of corporate financing and investment decisions: The financing present value ("FPV") approach to evaluating investment projects that change capital structure. Managerial Finance, 30(2), 16-37.
Woolley, S. (2009). Sources of value: A practical guide to the art and science of valuation. Cambridge: Cambridge University Press.