
NON-CONVENTIONAL PET NUCLIDES: PRODUCTION AND IMAGING
NUCLEIDOS DE PET NO CONVENCIONALES: PRODUCCIÓN Y PROYECCIÓN DE IMAGEN
Richard Laforest
Washington University School of Medicine (Mallinckrodt Institute of Radiology, St.Louis, USA)
Richard Laforest: laforestr@wustl.edu
(Recibido: Julio/2015. Aceptado: Septiembre/2015)
Abstract
Medical cyclotrons are now commonly used for the production of PET nuclides by the (pn) reaction. These devices are typically capable of delivering 10-15 MeV protons beams at sufficiently high intensity for timely production of β+ decaying nuclides. Non-conventional PET nuclides have emerged recently and offers new opportunities for diagnostic and therapy drug discovery. In this paper, we will review the production capabilities for such nuclides at Washington University Medical School in St. Louis and present their production. Finally, challenges for imaging imposed by the specific of the decay characteristics will be discussed.
Keywords: cyclotrons, PET Radionuclides, cascade gamma, positron range.
Resumen
Los ciclotrones médicos son usados actualmente para la producción de Nucleídos de PET. Esos dispositivos son capaces de producir haces de protones de 10-15 MeV con suficiente intensidad para la producción de radionúcleos con decaimiento β+. Los nucleídos no convencionales de PET han surgido recientemente y ofrecen nuevas oportunidades para diagnóstico y farmacoterapia. En este artículo se haría mención de la capacidad de producción de tales nucleídos en la Washington University Medical School. Serón discutidos los retos en imagenología impuestos por las características del decaimiento.
Palabras clave: Radionúcleos de PET, cascada de rayos gama, ciclotrón, rango de positrones.
Introduction
Radio-nuclides are widely used in medicine for imaging and radiotherapy applications. For imaging, β+ decaying nuclides are of special interest since the simultaneous emission of acolinear annihilation photons can be exploited to detect the localization of the spatial position of the activity. Contrary to single photon emission imaging, where the localization of the emission point is permissible only through the use of collimators, which themselves yields to significant reduction of sensitivity and spatial resolution, the coincident detection of the positron annihilation photons in PET (Positron Emission Tomography), allows for electronic collimation. This so called electronic collimation is responsible for the high sensitivity of PET cameras and thus high collection speed. These reasons have led to the high interest in PET for radiopharmaceuticals development for imaging and therapy, and thus the motivation for the wide interest in using this technology is to determine the spatial and temporal localization of imaging agents within the living human body.
PET of course started with the development of cameras that were initially used exclusively for bio-medical research in large institutions. The technology being inherently expensive, due to the cost of the machine but also due to the necessity of nearby cyclotrons. The advent of 18F-fluorodeoxyglucose (FDG), an 18F labeled glucose analog, propelled the use of this technology in the routine clinical arena in the 1990s and made this technology part of the standard of care of thousands of medical institutions in the world. The half-live of this nuclide (approximately 2hr) and the relatively low positron energy (average of 250 keV) are important factors that allowed the technology to grow. 18F is a pure β+ nuclide with a half-life ideal for radio-labeling, distribution and also in providing acceptable radiation dose to the patient.
FDG is an analog of glucose but unlike glucose, FDG is metabolized in a different way. FDG will be transported into the cells by the same mechanism as glucose but the labeling position of the 18F nuclide will prevent complete metabolization and will yield to trapping of the activity within the cells. Cancerous cells, being particular demanding in energy, will thus predominantly accumulate activity. FDG has thus become an essential tool in the armamentarium for the diagnosis, staging and evaluation or therapy effectiveness for many forms of cancers. Other nuclides have played a role in the early development of PET, such as 11C attached to a plethora of agents for various neuro-receptor studies, 15O-Water for cerebral blood flow analysis and 13NH3 cardiac perfusion and blood flow measurements. Those continue to be used intensively for PET imaging. These nuclides are now described as standard PET nuclides and are routinely produced with low energy medical cyclotrons. Some nuclides of common use can be produced by generators (e.g. 82Rb, 62Cu, 68Ga).
However those are not the only nuclides used for PET, many other proton rich nuclides can be used and those are of special interest for the development of novel radio-pharmaceuticals for diagnostic and radio-therapy applications and are an important part of the research being conducted at Washington University School of Medicine in St. Louis (USA). These so-called non-standard or non-conventional PET nuclides will be the subject of this presentation.
This chapter will present the production capabilities of radio-nuclides, describing the technology and some applications, notably for the imaging of novel imaging pathways that can shed light on the tumor biology itself. The document will focus on the presentation of the technology and will include a discussion of the imaging aspects with such nuclides. This chapter is the part of a three lectures series discussing the production of PET radio-nuclides. The second and third chapters will discuss the PET technology itself and the image reconstruction process while the last chapter will present the current status of the technology in research and clinical setting along with the current technical challenges and research opportunities being addressed.
Medical Isotopes from Cyclotrons
Medical cyclotrons consist typically of compact supra-conducting accelerating devices capable of producing proton and deuteron beams at approximately 15 MeV. Washington University School of Medicine in St.Louis currently operates 4 of these instruments. These include: the CS-15 (Cyclotron Corporation), JSW (Japan Steel Work), RDS-111 (Siemens), and the TR-19/9 (Advanced Cyclotron Systems). This one was installed over the summer of 2014 and will eventually replace the older CS-15 and JSW after their decommissioning. At 10-15 MeV, the most favorable method of production is from the production of excited compound nucleus by the (p,n), (p,α) or (d,n) reaction. The produced radio-nuclides remains essentially trapped in the target and need to be extracted by physical-chemistry methods.
Cu-64 Production
Cu-64 has tremendous interest at our institution and at many others in the world due to its favorable decay characteristics for PET imaging. The end point positron energy (identical to 18F) makes it an ideal PET nuclide for high-resolution and also its half-life of 12.7 hr allows for imaging up to 48 hr post injection. It is currently used for imaging the biodistribution of molecules targeting tumors. The combined β+ and β- makes this nuclide also of interest for radiotherapy applications due to the potential of delivering targeted therapy radiation doses. This nuclide is produced by the (p,n) reaction of electroplated 64Ni on a Au disk.
This nuclide is now in production in a weekly basis and we produce for distribution at more than 30 sites in the US. The recuperation of 64Cu proceeds by dissolving the nickel from the gold disk in 6M HCl and then passing the solution through an ion exchange column where both 64Ni and 64Cu can be recuperated[9].
Zr-89 Production
Zr-89 is produced from the (p,n) reaction on natural foil of 89Y and a Niobium target holder. The half-life of 3.27d is particularly well suited for studying the pharmacokinetics of antibodies that can have circulation time of 4-7 days within the body. It is thus a highly sought nuclide for immune-PET imaging which refers to evaluation of patient response to an antibody treatment and also evaluation for individualized patient dosing. The maximum beta energy of 0.9 MeV yield to an average positron range of only 1.2 mm which is not detrimental for PET imaging in clinical setting.
Beta decay proceed to the 9/2+ state of 89Y which has a 16 second half-life. This fortunate situation makes such that this nuclide appears essentially a pure β+ emitter[11].
Tc-94m Production
The interest of this nuclide is that it could provide an alternative to the highly popular 99mTc used for SPECT imaging. This nuclide would allow alleviating the limitations of SPECT in terms of sensitivity and resolution. This nuclide is produced via the (p,n) reaction on 94Mo and distillation proceeds through thermal diffusion at 1000 ?C with deposition of the activity on glass tube [6]. The problems with this nuclide are multiples: the maximum positron energy is rather high at 2.47 MeV (low resolution) but also numerous prompt gammas are emitter (high noise). In addition, 94gTc is co-produced which yield to a more complex decay correction[13]. Indeed, the measured activity being given by the sum of the two states can be represented by:
And thus, the 94mTc activity at any given time after end-of-bombardment (eob) is given by
Where, Am(t) and Ag(t) are the activity of the m and g state respectively, and λm, λg, Bm and Bg are the respective decay constant and beta decay branching ratios. These reasons have contributed to a declining interest in this nuclide. The alternate production strategies for 99mTc using accelerators or low-enriched uranium (LEU) reactors and improved SPECT camera design are other contributing factor to its demise.
Br-76 and 1-124 production
These nuclides are produced by the (p,n) reaction on 76Se or 124Te target[2, 14]. Since Se and Te are brittle, Cu2Se or Cu2Te targets can be used. Alternatively, 124TeO2 have been used. Target distillation proceeds by thermal distillation on a horizontal furnace capable of 1100 C and 1300 C under flow of N2. Activity is transported by the N2 gas and deposited on a Teflon tube. The long half-life of 124I makes production yield very low. Its long half-life makes this nuclide well suited for antibody imaging and since this nuclide is now available commercially, we no longer produce it on a routine basis.
In the next section we will discuss more closely the imaging capabilities of these nuclides in terms of decay characteristics and spatial resolution.
Imaging with Non-standard nuclides
Br-76 deserved some interest due to its decay characteristics. This nuclide decay by beta emission and electron capture to several excited states in 76Se. Notably, decay proceeds at approximately 40 % to excited states at approximately 3 MeV in 76Se. These states will then decay by gamma emission and will result in numerous cascade gamma rays with intense line at 559 keV (74%). Since the cascade gamma rays are emitted by the daughter nucleus with a very short half-live and the annihilation photons by the emitted positrons, the emission time difference will typically be shorter than the coincidence timing window of the PET scanner and there will be no angular correlation between the cascade gamma ray and the annihilation photons. This will yield to a PET event baring no spatial information on the localization of the activity. Considering that the positron-electron annihilation photons have a fixed energy of 511keV and that typical PET scanner have an energy resolution of 15-20 %, this prompt gamma will be undistinguishable from the annihilation photons and will yield to fortuitous coincidences that will affect image quality, quantitative accuracy and will increase image noise. Others nuclides such as 60Cu, 94mTc, 66Ga, 82Rb, 124I or 86Y are also notoriously known as "dirty" emitters and call for the application of techniques for correction or elimination for those spurious coincidences.
Cascade Removal Techniques
The most obvious consequence of these fortuitous coincidences from cascade gamma rays is by the appearance of a background of counts in projection space (not image space) which appear at a first approximation as uniform. This observation has thus prompted the implementation of simple uniform background subtraction in the sinograms[15, 16]. Beattie [17, 18] proposed a more elaborate deconvolution technique based on a uniform attenuation inside an ellipse fitted on the patient outline. Based on the observation that the Randoms coincidences have approximately the same distribution as the fortuitous cascade coincidences, it has been proposed that a simple scaling of the randoms distribution can be used. This approach offers very little computational cost and works reliably well for a source of activity not too close to the edge of the field of view. This approach has been implement by Siemens in their scanners. The drawbacks of this technique are that the random distribution is not always available and that this technique do not account for the attenuation of the cascades which may become an issue for large patients.
We proposed an alternative technique for which the cascade coincidence distribution is calculated as a similar fashion as the scatter distribution but for which no angular correlation exists between the cascade gamma ray and the annihilation photon[19]. This technique is based on the fact that the cascade coincidences are not random: they are simply true coincidences with no angular correlation and that their distribution can be exactly calculated knowing the approximate activity distribution and the attenuation image. The drawback is of course the increase computation time.
Indeed, the cascade distribution (in sinogram space) can be calculated by the following volume integral:
Where A(x, y, z) is the activity concentration at the location 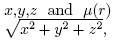 is the attenuation coefficient at location r = with ρ, θ,z the sinogram projection bin, angle and axial position respectively. This technique allows calculating the functional shape of the distribution, not its magnitude. The distribution can be scaled to the measured sinogram projection profiles by minimizing the function where α and β are scale factors for the Scatter and Cascade distributions.
is the attenuation coefficient at location r = with ρ, θ,z the sinogram projection bin, angle and axial position respectively. This technique allows calculating the functional shape of the distribution, not its magnitude. The distribution can be scaled to the measured sinogram projection profiles by minimizing the function where α and β are scale factors for the Scatter and Cascade distributions.
The technique result is presented in fig. 3 which shows a projection line profile through a uniform 6 cm diameter 76Br phantom, taken from [19].
Image Resolution
Essentially five parameters affect the observed spatial resolution in PET, two of them relates to the fundamental physics of positron decay. These parameters are detector crystal size, crystal penetration and inter-crystal scatter, annihilation photon non-acolinearity, positron range and finally the effects of reconstruction algorithm and the choice of the reconstruction parameters. Most current PET scanners employ the geometry of block detectors arranged in rings perpendicular to the imaging subject. The smallest detection elements is defined by the size of the scintillation crystal within a block and this size defines the sampling distance. Most manufacturers will use interleaving which essentially allows to use a sampling distance at half the crystal-crystal distance. Therefore, the crystal size contribution to the spatial resolution can be represented by a triangular response with a width at half the crystal size. The crystal penetration and inter-crystal scatter refers to the penetration of the gamma ray through multiple layers of scintillation material and scatter within a block. This effect is more difficult to calculate but can be calculated from Monte-Carlo simulations or by the use of an empirical "block-effect" factor. This factor is particularly spatially variant and will be responsible for the depth-of-interaction effect and the loss of resolution outside the central portion of the field-of-view. The basic physics of positron decay contribution of photon non-acolinearity can be modelled by a Gaussian distribution with σ = 0,022D, with D the scanner diameter (in cm). Finally, the positron range blurring effect is strongly dependent on the end-point positron energy as well as the specific of the decay characteristics. Indeed, for nuclides decaying to highly excited states in the daughter nucleus, the end-point energy will thereby be reduced and yield to lower positron energy and shorter range distribution. Finally, the image reconstruction algorithm (and its parameters) can have a strong influence on the measured spatial resolution.
The measured spatial resolution is thus be calculated by the convolution of all these factors as described by [20-22] and results in a spatially variant spatial resolution that can be characterized by a single parameter. In [23], we had demonstrated however that the effective spatial resolution can be more accurately characterized by the sum of two Gaussian distributions where the first Gaussian represents the scanner contribution to the resolution and the second contribution, the contribution from the long positron range for some nuclides with especially high maximal positron energy.
Some specially designed image reconstruction algorithms which include a model of the scanner spatial resolution can in fact improve the measured spatial resolution and produce optimal image of the activity distribution with both improved resolution and reduced noise. Such algorithms will be topic of the next chapter on PET Image Reconstruction. In this next chapter, we will review the PET technology, principle and image reconstruction theory.
Conclusions
This chapter described the production capabilities of radio-nuclides at Washington University. The production capabilities include all standard PET nuclides and a wide array of non-standard positron emitters that can be used for PET. Equipped with 4 cyclotrons, our center can accommodate our local use in radio-nuclides and the production of some nuclides has a suitable half-life for distribution to any center in North America. We have also discussed the limitations in image quality from the effect of cascade gamma rays and loss of resolution due to the long positron range and proposed correction techniques. In the next chapter, we will expand on this topic by the discussion of resolution recovery image reconstruction algorithm than can account for the positron range.
Acknowledgments
I wish to express my intense gratitude to Dr. Suzanne Lapi for providing me the material on the Cu-64 and Zr-89 production as well as all the current non-standard production information.
References
[1] T. J. McCarthy, D. W. McCarthy, R. Laforest, H. M. Bigott, F. Wust, D. E. Reichert, M. R. Lewis, and M. J. Welch, AIP Conf. Proc. , 576 (2001), conference date: 1-5 Nov 2000.
[2] V. Tolmachev, A. Lovqvist, L. Einarsson, J. Schultz, and H. Lundqvist, Appl. Radiat. Isot. 49, 1537 (1998).
[3] D. J. Rowland, R. Laforest, T. J. Mccarthy, B. J. Hughey, and M. J. Welch, J. Labelled Compd. Rad. 44, S1059 (2001).
[4] J. Schmitz, Eur. J. Nucl. Med. Mol. Imaging , Suppl 1: S4 (2011).
[5] J. Yoo, L. Tang, T. A. Perkins, D. J. Rowland, R. Laforest, J. S. Lewis, and M. J. Welch, Nucl. Med. Biol. 32, 891 (2005).
[6] H. Bigott, R. Laforest, X. Liu, A. Ruangma, F. Wuest, and M. Welch, Nucl. Med. Biol. 33, 923 (2006).
[7] M. Lewis, D. E. Reichert, R. Laforest, W. H. Margenau, R. E. Shefer, R. E. Klinkowstein, B. J. Hughey, and M. J. Welch, Nucl. Med. Biol. 29, 701 (2002).
[8] D. W. McCarthy, L. Bass, P. Cutler, R. Shefer, R. Klinkowstein, P. Herrero, J. Lewis, C. Cutler, C. Anderson, and M. Welch, Nucl. Med. Biol. 26, 351 (1999).
[9] D. W. McCarthy, R. Shefer, R. Klinkowstein, L. Bass, W. Margeneau, C. Cutler, C. Anderson, and M. Welch, Nuc. Med. Biol. 24, 35 (1997).
[10] A. L. Vavere, R. Laforest, and M. J. Welch, Nucl. Med. Biol. 32, 117 (2005).
[11] A. L. Wooten, Appl. Sci. 3, 593 (2013).
[12] A. L. Wooten, B. Lewis, and S. Lapi, Appl. Radiat. Isot. 96, 154 (2015).
[13] M. Smith, M. Daube-Witherspoon, P. P.S., L. Szajek, R. Carson, J. Everett, S. Green, P. Territo, R. Balaban, S. L. Bacharach, and W. Eckelman, Med. Phys. 28, 36 (2001).
[14] S. M. Qaim, Radiochim Acta 95, 67 (2007).
[15] M. Lubberink, H. Schneider, M. Bergstrom, and H. Lundqvist, Phys. Med. Biol. 47, 3519 (2002).
[16] K. S. Pentlow, R. D. Finn, S. M. Larson, Y. E. Erdi, B. J. Beattie, and J. L. Humm, Clin. Positron Imaging 3, 85 (2000).
[17] B. J. Beattie, Med. Phys. 30, 2410 (2003).
[18] B. Beattie and K. Pentlow, J. Nucl. Med. 45(suppl), 411P (2004).
[19] R. Laforest and X. Liu, Phys. Med. Biol. 54, 1503 (2009).
[20] C. S. Levin and E. J. Hoffman, Phys. Med. Biol. 44, 781 (1999).
[21] C. S. Levin and E. Hoffman, Phys. Med. Biol. 45, 559 (2000).
[22] R. Laforest, D. Rowland, and M. Welch, IEEE Trans. Nucl. Sci. 49, 2119 (2002).
[23] X. Liu and R. Laforest, Nucl. Med. Biol. 36, 551 (2009).