DETERMINACIÓN DEL CALOR ISOSTÉRICO PARA PULPA DE PLÁTANO (musa paradisiaca) POR ISOTERMAS DE SORCIÓN
Palabras clave:
Propiedades físicas, contenido de humedad, calor isostérico. (es)Las isotermas de sorción para ser usadas en la simulación y diseño de procesos de secado y almacenamiento de alimentos deben ser representadas por modelos válidos dentro de las condiciones encontradas en la práctica industrial. Usando el modelo de Chung–Pfost y ajustado por desorción el calor isostérico neto y total para pulpa de plátano fueron determinados. El calor neto isotérico neto decreció con el contenido de humedad del producto variando desde 1670 kJ/kg (5%.d.b) a 215 kJ/kg (26%.d.b) donde esta variación fue representada por el modelo potencial y polinomial.
DETERMINATION OF THE ISOSTERIC HEAT TO PLANTAIN PULP (musa paradisiaca) BY SORPTION ISOTHERMS
DETERMINACIÓN DEL CALOR ISOSTÉRICO PARA PULPA DE PLÁTANO (musa paradisiaca) POR ISOTERMAS DE SORCIÓN
HÉCTOR
CIRO
Profesor, Departamento de Ingeniería Agrícola y de
Alimentos, Universidad Nacional de Colombia
JAIRO
ALEXANDER OSORIO
Profesor, Departamento de Ingeniería Agrícola y de
Alimentos, Universidad Nacional de Colombia
ELKIN
ALONSO CORTÉS
Profesor, Departamento de Ingeniería Agrícola y de Alimentos, Universidad Nacional
de Colombia, ecortes@unalmed.edu.co
Recibido para revisar Marzo 15 de 2008, aceptado Junio 18 de 2008, versión final Junio 26 de 2008
ABSTRACT: For sorptional data to be useful in simulation and design of storage and drying systems, they must be represented by equations valid in the conditions usually found in industrial practice. Using the modified Chung–Pfost model and fitted by desorption the net and total isosteric heat of plantain pulp was evaluated. The net isosteric heat decreased with increasing moisture content ranging from 1670 kJ/kg (5%.d.b) to 215 kJ/kg (26%.d.b) where the better goodness of fit was presented by polynomial and power-law models.
KEYWORDS: Physical properties, moisture content, isosteric heat.
RESUMEN: Las isotermas de sorción para ser usadas en la simulación y diseño de procesos de secado y almacenamiento de alimentos deben ser representadas por modelos válidos dentro de las condiciones encontradas en la práctica industrial. Usando el modelo de Chung–Pfost y ajustado por desorción el calor isostérico neto y total para pulpa de plátano fueron determinados. El calor neto isotérico neto decreció con el contenido de humedad del producto variando desde 1670 kJ/kg (5%.d.b) a 215 kJ/kg (26%.d.b) donde esta variación fue representada por el modelo potencial y polinomial.
PALABRAS CLAVE Propiedades físicas, contenido de humedad, calor isostérico.
1. INTRODUCTION
The plantain is a species of the genus Musa and is generally used for cooking, in contrast to the soft, sweet banana (which is sometimes called the dessert banana). Plantains tend to be firmer and lower in sugar content than dessert bananas and are used either when green or under-ripe (and therefore starchy) or overripe (and therefore sweet). Plantains are a staple food in the tropical regions of the world, treated in much the same way as potatoes and with a similar neutral flavour and texture when unripe. In Colombia the plantain under-ripe is called as “ plátano verde” and its pulp is used extensively by its high culinary quality and industrial processing as flour. The “plátano” is one of the products more important at domestic marketing, since it participates with the 6,8% of the agricultural production occupying the fifth place after the coffee, flowers, banana and sugarcane. This product is fundamental in the Colombians diet with a consumption by person of 61,9 kg/year.
The sorption isosteric heat (also called latent heat of vaporization, desorption–vaporization) in foodstuffs is of practical interest in drying operations, handling, storage and processing (Mulet et al., 2002; Ashraful et al., 2007; Yan et al., 2008). The value of latent heat of vaporization is neither constant nor equal to the heat of pure water evaporation (Tolaba et al., 2004). It is a function of temperature and moisture content, that is, the value is varied throughout the drying process (Rucklod et al., 2003). A simple model including moisture content and temperature term is very useful to compute the latent heat of vaporization (Kaya and Kahyaoglu, 2005).
The net isosteric heat is defined as the total heat of sorption in the food minus the heat of vaporization of water, at the system temperature (Tsami, 1990). The total isoteric heat of sorption is the total heat supply for drying of a material. As a differential molar quantity, isosteric heat of sorption determines the temperature dependence of water activity of a biological material. The heat of vaporization of sorbed water may increase to values well above the vaporization of pure water as food is dehydrated to low moisture levels (Rizvi, 2005). The extent of material moisture content at which the isosteric heat of sorption approaches the latent heat of vaporization of water is often considered as an indication of the amount of “bound water” existing in the food (Kiranoudis et al., 1993). Conventionally, is a positive quantity when heat is evolved during adsorption, and negative when heat is absorbed during desorption. The heat of adsorption is a measure of the energy released on sorption, and the heat of desorption the energy requirement to break the intermolecular forces between the molecules of water vapour and the surface of adsorbent. Thus, the heat of sorption is considered as indicative of the intermolecular attractive forces between the sorption sites and water vapour (Rizvi, 2005).
The application of thermodynamic principles to sorption isotherm data has been used to obtain more information about the properties of water, food microstructure, and physical phenomena on the food surfaces, and sorption kinetic parameters (McMinn and Magee, 2003). The net isosteric heat of sorption is used as thermodynamic function for analysis of sorption isotherms. The net isosteric heat of sorption or differential enthalpy shows the energy requirement for removing moisture from food material (water–solid binding strength) has a practical use in complete drying calculations and modeling of energy (Rizvi, 2005). The net integral enthalpy or net equilibrium heat of sorption indicates the binding strength of water molecules to food particles and could be a measure of the food–water affinity (Aviara et al., 2004).
Two methods are available for measurement of the differential heat of sorption. The first is direct calorimetric measurement of the heat evolved, and the second is application of the Clausius-Clayperon equation on the isosteric equilibrium pressures at different temperatures (the ‘isosteric’ heat of sorption). Sorption calorimetry is difficult because of the technique needed for precise measurement of the small quantities of heat evolved. For this reason, calorimetrical measured heats of sorption are much less common than those calculated from the sorption isotherm, however, they offer a higher degree of accuracy when determined with care (Al-Muhtaseb et al., 2002).
Moisture sorption isotherms describe the relationship between the equilibrium moisture content and the water activity at constant temperatures and pressures (Kaleemullah and Kailappan, 2004). For food materials these isotherms give information about the sorption mechanism and the interaction of food biopolymers with water. The moisture sorption isotherms are extremely important in modelling the drying process, in design and optimisation of drying equipment, in predicting shelf-life stability, in calculating moisture changes which may occur during storage and in selecting appropriate packaging material (Gabas et al., 2000; Kaymak-Ertekin and Gedik, 2004)
The objective of this study was to determine the thermodynamic function of isosteric heat in relation to moisture sorption in plantain pulp using a sorption model.
2. MATERIALS AND METHODS
Isotherm models
In this study the isosteric heat of plantain pulp was determined through the
sorption isotherm method. Two Aw models incorporating temperature terms have been evaluated for sorption data (Ciro et
al., in press). The modified Chung–Pfost model fitted by desorption was adopted in this study to compute the qst (the net isosteric heat) and Qst (total heat of
sorption) values. The fitted model is:
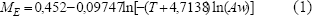
The model cited above presented the best goodness of fit (Ciro et al., in press) and was fitted to 25ºC≤T≤50ºC, activity water of 0,4≤Aw≤0,8 and equilibrium moisture content of 5% d.b≤ ME ≤ 26%d.b.
Net isosteric
heat of sorption The Clausius–Clapeyron
equation
The net isosteric heat of sorption (qst) is obtained by subtraction
of heat of water vaporization from total heat of sorption (Qst). The qst could be determined from experimental data using Clausius- Clayperon
equation in the form(Aguerre et al., 1988; Rizvi, 2005; Chen, 2006;
Perez-Alonso et al., 2006; Vullioud et al., 2006; Samapundo et al., 2007):

Integrating Eq. (2), assuming that the net isosteric heat of sorption (qst) is temperature independent gives the following equation:
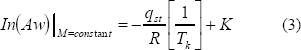
Where:
qst= Qst-l (kJ/kg)
K= Integration constant
Aw= Water activity (decimal)
l= Latent heat of vaporization of pure water (kJ/kg)
R= Gas constant (0,4618 kJ/kg K)
Tk= Temperature (K)
M= Moisture content (% dry basis)
The heat to vaporization of pure water (l) can be calculated by:
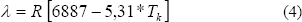
In this study, the isosteric heat of sorption was determined from the slope after plotting ln(Aw) versus 1/TK at constant moisture content. This approach assumes that isosteric heat of sorption does not change with temperature.
The Othmer
equation
The relationship between Pvs (saturated water vapor pressure) and the Pv (vapor pressure of water in food products) was given by Othmer (1940) as:
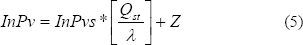
where l is the heat of evaporization for pure water in kJ/kg, Qst total heat of sorption isosteric or latent heat of vaporization of food products in kJ/kg (Brooker et al., 1992); P v is the vapor
pressure in food stuffs in kPa; and Pvs is the saturate vapor pressure in kPa. This model considers that the Qst/l value is independent of temperature. From the linear regression analysis technique, the slope, Qst, can be found from ln(Pv) and ln(Pvs) data.
In the equation (5), the saturation vapor pressure was determined using the Tetens equation (Weisss, 1977):

The vapor pressure in the food was calculated as:

To equilibrium conditions the equilibrium relative humidity (ERH) is equal to water activity (Aw) (Jayendra et al., 2005; Ashraful et al., 2007)
Fitting Models
Several researchers reported the isosteric heat of sorption as an empirical function of
moisture content (Tsami et al., 1990; Wang and Brennan, 1991; Sopade and Ajisegiri, 1994;Oztekin and Soysal, 2000; Hossain et al.,2001; Kaya and Kahyaoglu, 2005 Chen, 2006; Ashraful et al., 2007; Iguaz and Vírseda, 2007). The isosteric heat of sorption of plantain pulp as a function of equilibrium moisture content (ME) in the following forms were considered:

In the equation (8), “b” indicates the changes in binding energy with changes in water content, and “a” is the isosteric heat of sorption for the strongest bound water molecule (Rahman et al., 2002).
The accuracy of fit was evaluated by calculating the root mean square percent error (RMS) (Lievonen and Roos, 2002):

Uexp and Up are the values of isosteric heat observed and predicted, respectively.
3. RESULTS AND DISCUSSION
Isosteric
heat of sorption
Values of the heat of sorption at a specific
moisture content provides an indication of the state of the sorbed water and
hence, a measure of the physical, chemical and microbiological stability of the
food material under given storage conditions. In addition, the variation in
heat of sorption with moisture content provides valuable data for energy
consumption calculations and subsequent design of drying equipment, and
knowledge of the extent of the water–solid versus water–water interactions
(McMinn and Magee, 2003). The net isosteric heats of sorption values with respect to moisture
content are represented in Fig. 1. The curve shows that the heat of desorption
decreased with increase in moisture content, initially rapidly up to 15 g moisture/100 g drysolids
and later slowly, as observed in many other food systems (Sawhney et al., 1991; Tsami, 1991; Rahman et al., 2002; Delgado and Sun, 2002b; Tolaba et al., 2004; Yazdani et al., 2006; Sapamundo
et al., 2007; Ashraful
et al., 2007; García-Pérez et al., 2008). The decrease in the isosteric heat with the
increase in sorbed water content may be attributed to the fact that sorption
initially occurs on the most active sites such as hydrophilic polar groups
giving rise to the greatest interaction energy. With increase in the moisture
content, as these sites become occupied, sorption occurs on the less active
sites viz., peptide bonds or hydrophobic hydration sites resulting in lower heats
of sorption (Delgado and Sun, 2002b; Jayendra et al., 2005).

Figure 1. Net isosteric heat as a
function of equilibrium moisture content (equation 3)
The ratio of latent heat of vaporization as a function of moisture content calculated by Othmer equation can be observed in Fig. 2. The ratio of (Qst/l) at low moisture contents is high, indicating that the heat of sorption is nearly twice the heat of vaporization of pure water (l). This initial heat may result probably from the chemisorption on polar groups (Simal et al., 2007). The value of (Qst/l) was higher than one at every moisture contents but tending to one as the moisture content increased. The results of the Fig. 1 shown that during a drying process of plantain pulp the energy requirements are very high.
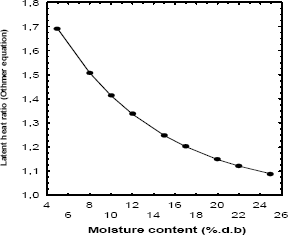
Figure 2. Latent heat of vaporization using
Othmer´s equation
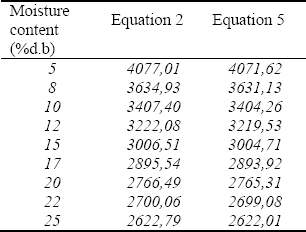
The analysis statistical (ANOVA with P=0,05) did not found differences to the total isosteric heat calculated by Clausius–Clapeyron and Othmer equation. The total isosteric heats of desorption of plantain pulp are presented in Table 1. It is evident a marked increases in isosteric heat at the lower moisture contents. The evaporation of water from pulp requires energy to overcome the heat of evaporation of pure water (2408,4 kJ/kg). The values were calculated to 25ºC using the Eq. 2 and 5.
Table 1. Total isosteric heat as a function of moisture content to plantain pulp
Fitting Models
The calculation of isosteric heat variation with
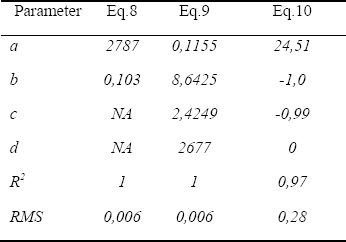
moisture content was done using the power model (Eq.8), polynomial model (Eq.9)
and non-linear model (Eq.10) and plotted in Fig. 3. The agreement was excellent
for the desorption curve (R2@1). The parameters and the standard error of estimate of the parameters
for fitting the Eq. (8), (9) and (10) are
shown in Table 2. The results of Table 2 suggesting that polynomial and power model
considered in this study can be used to predict the net isosteric heat of plantain pulp with excellent approximation.

Figure 3. Fitted models to net isosteric
heat according to moisture content
Table 2. Estimated parameters for the different models
4. CONCLUSION
The net and total isosteric heat of sorption by desorption of plantain pulp increased with decreasing moisture content. The net isosteric heat was found to be a polynomial and power function of equilibrium moisture content. This set of equations will be useful in the simulation of drying and storage of plantain pulp.
REFERENCES
[1] AGUERRE, R. J; SUAREZ C. AND VIOLLAZ, P. E. The temperature dependence of isosteric heat of sorption of some cereal grains, International Journal of Food Science and Technology, 23, 141-145, 1988.
[2] AL-MUHTASEB, A. H., MCMINN, W. A. M. AND MAGEE, T. R. A., Moisture sorption isotherm characteristics of food products: a review, Trans IChemE, 80, 118-128, 2002.
[3] ASHRAFUL HAQUE, MD., SHIMIZU, NAOTO AND KIMURA, TOSHINORi, Net isosteric heats of adsorption and desorption for different forms of hybrid rice, International Journal of Food Properties, 10, 25–37, 2007.
[4] AVIARA, N. A AND AJIBOLA, O. O., Thermodynamics of moisture sorption in melon seed and cassava. 2002. Journal of Food Engineering, 55, 107–113, 2002.
[5] BROOKER, D.B., BAKER-ARKEMA, F.W AND HALL, C.W. Drying and Storage of Grains and Oilseeds, The AVI Publishing COMPANY, NEW YORK, 1992.
[6] CHEN C., Obtaining the isosteric sorption heat directly by sorption isotherm equations, Journal of Food Engineering, 74, 178–185, 2006.
[7] DELGADO, A. E., AND SUN, D.-W., Desorption isotherms for cooked and cured beef and pork, Journal of Food Engineering, 51, 163–170, 2002b.
[8] GABAS A.L., MENEGALLI, F.C., AND TELIS-ROMERO J., Water sorption enthalpy-entropy compensation based on isotherms of plum skin and pulp, Journal of Food Science, 65 (4), 680-684, 2000.
[9] GARCÍA-PÉREZ, J.V., CÁRCEL, J.A., CLEMENTE, G. AND MULET, A. Water sorption isotherms for lemon peel at different temperatures and isosteric heats, LWT , 41, 18–25, 2008.
[10] HOSSAIN, M.D., BALA, B.K., HOSSAIN, M.A., MONDOL, M.R.A., Sorption isotherms and heat of sorption of pineapple, J. Food Eng., 48 (2), 103–107, 2001.
[11] IGUAZ, A. AND VÍRSEDA, P., Moisture desorption isotherms of rough rice at high temperatures, Journal of Food Engineering 79, 794–802, 2007.
[12] JAYENDRA KUMAR, A., SINGH, R.R.B., PATIL, G.R AND PATEL, A.A., Effect of temperature on moisture desorption isotherms of kheer, LWT, 38, 303–310, 2005.
[13] KALEEMULLAH, S. AND KAILAPPAN, R. Moisture sorption isotherms of red chillies, Biosystems Engineering, 88 (1), 95–104, 2004.
[14] KAYA, S AND KAHYAOGLU, T, Thermodynamic properties and sorption equilibrium of pestil (grape leather), Journal of Food Engineering , 7, 200–207, 2005.
[15] KAYMAK-ERTEKIN, FIGEN AND GEDIK, ATIL, Sorption isotherms andisosteric heat of sorption for grapes, apricots, apples and potatoes, Lebensm.-Wiss. u.-Technol., 37,429–438, 2004.
[16] KIRANOUDIS, C.T., MAROULIS, Z.B., TSAMI, E., MARINOS-KOURIS, D., Equilibrium moisture content and heat of desorption of some vegetables, J. Food Eng., 20 (1), 55–74, 1993.
[17] LIEVONEN, S.M. AND ROOS, Y.H. Water sorption of food models for studies of glass transition and reaction kinetics, Journal of Food Science, 67(5),1758-1766, 2002.
[18] MCMINN, W. A. M AND MAGEE, T. R. A., Thermodynamic properties of moisture sorption of potato, Journal of Food Engineering, 60, 155–157, 2003.
[19] MULET, A., GARCIA-PASCUAL, P., SANJUÁN, N AND GARCÍA-REVERTER, J., Equilibrium isotherms and isosteric heats of morel (Morchella esculenta), Journal of Food Engineering, 53,75–81,2002.
[20] OTHMER DF., Correlating vapor pressure and latent heat data. A new plot, Ind Eng Chem, 32:841-856, 1940.
[21] OZTEKIN, S. AND SOYSAL, Y., Comparison of Adsorption and Desorption Isosteric Heats for Some grains. Agricultural Engineering International: the CIGR Journal of Scientific Research and development 2000, II, 1–17.
[22] PÉREZ-ALONSO, C.; BERISTAIN, C.I.; LOBATO-CALLEROS, C.; RODRÍGUEZ-HUEZO, M.E. AND VERNON-CARTER, E.J., Thermodynamic analysis of the sorption isotherms of pure and blended carbohydrate polymers, Journal of Food Engineering, 77, 753–760, 2006.
[23] RAHMAN, M. S., SABLANI, S. S., AL–RUZEIQI, GUIZANI, M. H., N., Water adsorption isotherms of freeze–dried tuna meat, Transactions of the ASAE, 45(3), 767–772, 2002.
[24] RIZVI,S,S,H., Thermodynamics properties of foods in dehydration, In: Engineering properties of food (Third Edition), Editor: M. A. Rao; Syed S.H. Rizvi and Ashim K. Datta, New York: CRC Press, 239-326, 2005.
[25] RUCKLOD, S., ISENGARD, H.-D., HANSS, J., AND GROBECKER, K. H., The energy of interaction between water and surfaces of biological reference materials, Food Chemistry, 82, 51–59, 2003.
[26] SAMAPUNDO, SIMBARASHE, DEVLIEGHERE, FRANK, DE MEULENAER, BRUNO, ATUKWASE, ABEL, LAMBONI, YENDOUBAN AND DEBEVERE, JOHAN M., Sorption isotherms and isosteric heats of sorption of whole yellow dent corn, Journal of Food Engineering, 79, 168–175, 2007.
[27] SAWHNEY, I.K., PATIL, G.R. AND BIKRAM KUMAR. Effect of temperature on moisture sorption isotherms of heat treated whole milk product khoa, Journal of Dairy Research, 58, 329-335, 1991.
[28] SIMAL, SUSANA, FEMENIA ANTONI, CASTELL-PALOU, AFRICA, ROSSELLO, CARMEN , Water desorption thermodynamic properties of pineapple, Journal of Food Engineering, 80, 1293–1301, 2007.
[29] SOPADE, P.A. AND AJISEGIRI, E.S. Moisture sorption study on Nigerian foods: maize and sorghum, Journal of Food Engineering, 17(1), 33-56, 1994.
[30] TOLABA, MARCELA P., PELTZER, MERCEDES, ENRIQUEZ, NATALIA AND POLLIO MARÍA LUCÍA, Grain sorption equilibria of quinoa grains, Journal of Food Engineering, 61,365–371, 2004.
[31] TSAMI E., Net isosteric heat of sorption in dried fruits, J. Food Eng. , 14, 327–335, 1991.
[32] TSAMI, E., MAROULIS, Z. B., MORUNOS-KOURIS,D. AND SARAVACOS, G. D., Heat of sorption of water in dried fruits, Int J Food Sci Technol, 25: 350–359, 1990.
[33] VULLIOUD, M.; MÁRQUEZ, C.A AND DE MICHELIS, A., Equilibrium sorption isotherms and isosteric heat of rose hip fruits (rosa eglanteria), International Journal of Food Properties, 9, 823–833, 2006.
[34] WANG, N. AND BRENNAN, J.G., Moisture Sorption Isotherm Characteristics of Potatoes at four temperatures, J. Food Eng. , 14 (4), 269–287, 1991.
[35] WEISSS, A., Algorithms for the calculation of moist air properties on a hand calculator, Trans ASAE, 20, 1133-1136, 1977.
[36] YAN, ZHENGYONG., SOUSA-GALLAGHER, MARIA J AND OLIVEIRA, FERNANDA A.R., Sorption isotherms and moisture sorption hysteresis of intermediate moisture content banana, Journal of Food Engineering, 86, 342–348, 2008.
[37] YAZDANI, M., SAZANDEHCHI P., AZIZI, M AND GHOBADI, P., Moisture sorption isotherms and isosteric heat for pistachio, European Food Research and Technology, 223(5), 577-584, 2006.
Cómo citar
IEEE
ACM
ACS
APA
ABNT
Chicago
Harvard
MLA
Turabian
Vancouver
Descargar cita
Visitas a la página del resumen del artículo
Descargas
Licencia
Derechos de autor 2008 DYNA

Esta obra está bajo una licencia internacional Creative Commons Atribución-NoComercial-SinDerivadas 4.0.
El autor o autores de un artículo aceptado para publicación en cualquiera de las revistas editadas por la facultad de Minas cederán la totalidad de los derechos patrimoniales a la Universidad Nacional de Colombia de manera gratuita, dentro de los cuáles se incluyen: el derecho a editar, publicar, reproducir y distribuir tanto en medios impresos como digitales, además de incluir en artículo en índices internacionales y/o bases de datos, de igual manera, se faculta a la editorial para utilizar las imágenes, tablas y/o cualquier material gráfico presentado en el artículo para el diseño de carátulas o posters de la misma revista.
















