Published
Desarrollo y validación de una metodología analítica por HPLC para la cuantificación de fenobarbital en una suspensión extemporánea
Keywords:
fenobarbital, suspensión extemporánea, validación, hplc, arreglo de diodos (es)Downloads
Estimation of the indomethacin solubility in ethanol + water mixtures by the extended Hildebrand solubility approach
Método extendido de Hildebrand en la estimación de la solubilidad de la indometacina en mezclas etanol + agua
Miller A. Ruidiaz 1 , Daniel R. Delgado 1 , Carolina P. Mora 2 , Alicia Yurquina3 , Fleming Martínez 1 *
1 Grupo de Investigaciones Farmacéutico-Fisicoquímicas, Departamento de Farmacia, Facultad de Ciencias, Universidad Nacional de Colombia, A. A. 14490, Bogotá D. C., Colombia. *Corresponding author e-mail: fmartinezr@unal.edu.co
2 Departamento de Química Farmacéutica, Facultad de Ciencias Naturales, Universidad ICESI, Santiago de Cali, Colombia.
3 Instituto de Química Inorgánica, Facultad de Bioquímica, Química y Farmacia, Universidad Nacional de Tucumán, San Miguel de Tucumán, Argentina.
Recibido para evaluación: March 31, 2010 Aceptado para publicación: May 17, 2010
RESUMEN
Indomethacin (IMC) is an analgesic drug whose physicochemical properties have not been thoroughly studied. In this work the Extended Hildebrand Solubility Approach (EHSA) was applied to evaluate the solubility of IMC in ethanol + water mixtures at 298.15 K. An acceptable correlative capacity of EHSA was found using a regular polynomial model in order four (overall deviation lower than 4.1%), when the W interaction parameter is related to the solubility parameter of the mixtures. Besides, the deviations obtained in the estimated solubility with respect to experimental solubility were lower compared with those obtained directly by means of an empiric regression of the experimental solubility as a function of the mixtures´ solubility parameters.
Key words: Binary mixtures, Extended Hildebrand Solubility Approach, Indomethacin, Solubility parameter.
RESUMEN
La indometacina (IMC) es un analgésico cuyas propiedades fisicoquímicas aún no han sido totalmente estudiadas. En la presente investigación, se aplicó el Método Extendido de Solubilidad de Hildebrand (MESH) al estudio de la solubilidad de la IMC en mezclas binarias etanol + agua a 298,15 K. Se obtuvo una capacidad predictiva aceptable del MESH (desviación general inferior al 4,1%) al utilizar un modelo polinómico regular de cuarto orden relacionando el parámetro de interacción W con el parámetro de solubilidad de las mezclas solventes. De esta forma, las desviaciones obtenidas en la solubilidad estimada, fueron de magnitud inferior a las obtenidas al calcular esta propiedad directamente, utilizando una regresión empírica regular del mismo orden, de la solubilidad experimental del fármaco en función del parámetro de solubilidad de las mezclas disolventes.
Palabras clave: Indometacina, Método Extendido de Solubilidad de Hildebrand, Mezclas binarias, Parámetro de solubilidad.
INTRODUCTION
Indomethacin (IMC, Fig. 1) is a non-steroidal anti-inflammatory drug used as analgesic and antipyretic, among other indications (1, 2). In the Colombian market this drug is available as capsules, oil ophthalmic drops, and injectable powder for reconstitution intended to intramuscular administration, but it is not available as any homogeneous liquid dosage form (3). Although IMC is used in therapeutics, the physicochemical information about its solubility is not abundant. On this way, it is well known that several physicochemical properties such as, the solubility of active ingredients and excipients close to the respective occupied volumes in useful solutions, are very important for the pharmaceutical scientist, because they facilitate the processes associate to design and development of new products in the pharmaceutical industries (4). In view of the facts, there is considerable scope for the study the behavior of IMC in hydroalcoholic solutions. Taking into account that IMC is practically insoluble in water by the low polarity in comparison with that, the hydroalcoholic solution reduces the polarity of the medium and is expected then an increase of IMC solubility.
For these reasons, this report presents a physicochemical study about the solubility prediction of IMC in binary mixtures conformed by ethanol and water. The study was done based on the Extended Hildebrand Solubility Approach (EHSA), developed by Martin et al. to use it in pharmaceutical systems (5-7). As has been already described, the solubility behavior of drugs in cosolvent mixtures is very important because cosolvent blends are frequently used in purification methods, preformulation studies, and pharmaceutical dosage forms design, among other applications (8, 9). This report expands the information presented about the solubility prediction of other analgesic drugs by means of EHSA method (10-14) including the one developed recently for this drug in ethyl acetate + ethanol mixtures (15).
THEORETICAL
The ideal solubility ( Xid2 ) of a solid solute in a liquid solution is calculated adequately by means of the expression,

where, ÎH fus is the fusion enthalpy of the solute, R is the universal gas constant (8.314 J mol -1 K -1 ), T fus is the melting point of the solute, and T is the absolute temperature of the solution. On the other hand, the real solubility (X 2 ) is calculated by adding the non-ideality term, (log γ 2 ), to equation 1 (16, 17), in order to obtain the following expression,

where, V 2 is the partial molar volume of the solute (cm 3 mol -1 ),Φ 1 is the volume fraction of the solvent in the saturated solution, and δ 1 and δ2 are the solubility parameters of solvent and solute, respectively. The solubility parameter,δ , is calculated as ((ÎHv-RT)/V1)1/2 , where, ÎH v is the vaporization enthalpy and V l is the molar volume of the liquid.
The vast majority of pharmaceutical dissolutions deviate notoriously of that predicted by the regular solutions theory (because of the strong interactions present, such as hydrogen bonding, in addition to the differences in molar volumes among solutes and solvents). On this way, at the beginning of the 80s of the past century, Martin et al. developed the EHSA method, which has been useful to estimate the solubility of several drugs in binary and ternary cosolvent systems (5-7). Accordingly, if the A term (defined as V2Φ21/(2.303 RT)) is introduced in theequation 3, the real solubility of drugs and other compounds in any solvent can be calculated from the expression,
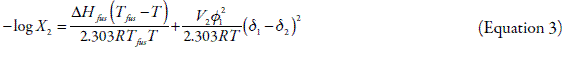

where, the W term is equal to 2Kδ 1δ 2 (where, K is the Walker parameter, Ref. 16). The W factor compensates the deviations observed with respect to the behavior of regular solutions, and it can be calculated from experimental data by means of the following expression,

where, γ 2 is the activity coefficient of the solute in the saturated solution, and it is calculated as the quotient, Xid2/X2
The experimental values obtained for the W parameter can be correlated by means of regression analysis by using regular polynomials in superior order as a function of the solubility parameter of the solvent mixtures, as follows,

These empiric models can be used to estimate the drug solubility by means of back calculation resolving this property from the specific W value obtained in the respective polynomial regression.
EXPERIMENTAL
Reagents
Indomethacin [1-(4-Chlorobenzoyl)-5-methoxy-2-methyl-1H-indole-3-acetic acid, CAS: 53-86-1] used was in agreement with the quality requirements indicated in the British Pharmacopoeia, BP (19). In similar way, absolute ethanol A.R. Merck (EtOH), distilled water with conductivity < 2 mS cm -1 , and Millipore Corp. Swinnex®-13 filter units, were also used.
Solvent mixtures preparation
The dehydrated ethanol employed was maintained over molecular sieve (Merck Number 3, 0.3 nm in pore diameter) to obtain a dry solvent previously to prepare the solvent mixtures. The ethanol dryness was demonstrated by the respective density value obtained (0.7854 g cm -3 at 298.15 K), which was thus coincident with those reported in the literature (20, 21). All EtOH + water solvent mixtures were prepared in quantities of 50.00 g by mass using an Ohaus Pioneer TM PA214 analytical balance with sensitivity ñ 0.1 mg, in mass fractions from 0.10 to 0.90 varying by 0.10, in order to study nine mixtures and both pure solvents.
Solubility determination
An excess of IMC was added to each cosolvent mixture evaluated in stoppered dark glass flasks. Solid-liquid mixtures were placed on a thermostatic bath (Neslab RTE 10 Digital One Thermo Electron Company) kept at 298.15 ñ 0.05 K with sporadic stirring for at least seven days to reach the saturation equilibrium (this equilibrium time was established by quantifying the IMC concentration up to obtain a constant value). In the case of neat water or water-rich mixtures the equilibration time was 14 days. Once at equilibrium, supernatant solutions were filtered (at isothermal conditions) to remove insoluble particles before composition analysis. IMC concentrations in neat EtOH and the EtOH + W mixtures up to 0.40 in mass fraction of water were determined by mass balance by weighing a specified quantity of the respective saturated solutions and allowing the solvent evaporation up to constant masses. In the other hand, IMC concentrations in all the other systems studied (from 0.50 in mass fraction of water to pure water) were determined by measuring UV-absorbance after appropriate gravimetric dilutions with ethanol and interpolation from a previously constructed UV spectrophotometric calibration curve (UV/VIS BioMate 3 Thermo Electron Company spectrophotometer). In order to make the equivalence between volumetric and gravimetric concentration scales, the density of the saturated solutions was determined with a digital density meter (DMA 45 Anton Paar) connected to the same recirculating thermostatic bath.
Estimation of the volumetric contributions
Because the equations 3 to 5 require the volume contributions of each component to the saturated solution, in this investigation the IMC apparent specific volume (ΦV spc ) was used to calculate these contributions. The ΦVspc values were calculated according to equation 7 (22),

where, m 2 and m 1 are the masses of solute and solvent in the saturated solution, respec- tively, VE 1 is the specific volume of the solvent, and Ρ soln is the solution density. The IMC apparent molar volume is calculated by multiplying the ΦV spc value and the molar mass of the solute (357.8 g mol -1 , Ref. 1). Otherwise, the calculated molar volume value obtained by means of the Fedors method was used in the later calculations and it was taken from the literature (230.0 cm 3 mol -1 , Ref. 15).
RESULTS AND DISCUSSION
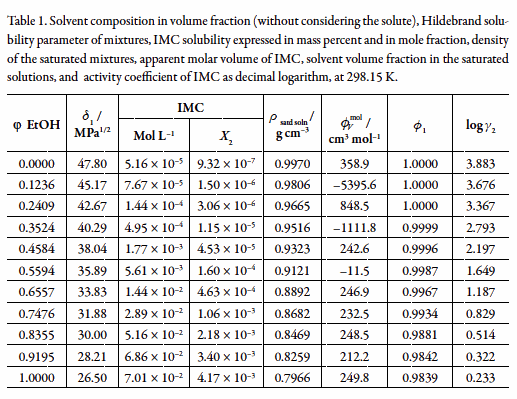
The information about polarity and volumetric behavior of EtOH + water mixtures as a function of the composition is shown in Table 1 and it was taken from the literature (13, 14, 23). It is important to note that the solubility parameter values of the solvent medium are in good agreement with those obtained from experimental permittivity data (24), which were thus calculated by using the Paruta equation (25). On the other hand, the calorimetric values reported in the literature for IMC were as follows, T fus = 432.6 K and ÎH fus = 39.46 kJ mol -1 . (26). From these values the calculated ideal solubility for this drug was 7.123 x 10 -3 in mole fraction (15).
Table 1 also summarizes the IMC solubility expressed in molarity and mole fraction, the density of the saturated mixtures, the apparent molar volume of IMC, and the sol- vent volume fraction in the saturated solutions at 298.15 K. Figure 2 shows the experimental solubility and the calculated solubility by using the regular solution model (Equation 3) as a function of the solubility parameter of solvent mixtures.
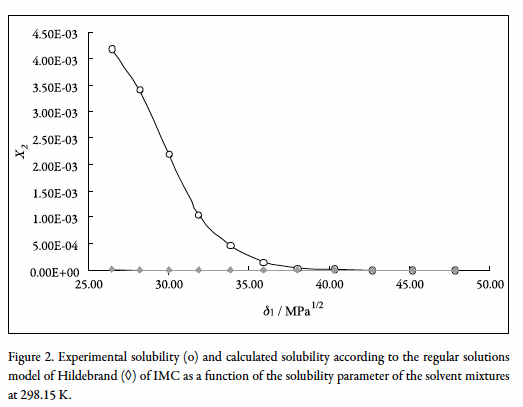
The IMC solubility is greater in EtOH but this value is lower than the obtained in the mixture of 0.6720 in volume fraction of ethyl acetate in mixtures conformed by ethyl acetate + EtOH (whose mixture has δ 1 = 20.86 MPa 1/2)(15).
From density values of cosolvent mixtures (23) and saturated solutions (Table 1), in addition to IMC solubility (Table 1), the solvent volume fraction (Φ 1 ) and apparent molar volume of the solute (ΦV mol) in the saturated mixtures, were calculated. These values are also presented in Table 1.
In the literature (16, 17), the solute molar volume in the saturated solution has been considered as a constant value when EHSA method is used. On this way, for solid compounds this property is generally calculated by means of groups´ contribution methods such as developed by Fedors (27). Nevertheless, this property is not independent on the solvent composition as can be see in Table 1 for apparent molar volume of IMC. This fact would be due to the different intermolecular interactions, depending on the respective solvent proportions. Nevertheless, the experimental values are variable and unclear, in particular for water-rich mixtures, where negative values were obtained. For this reason, in this investigation the calculated molar volume (230.0 cm 3 mol-1 , Ref. 15) was employed in the following calculations.
On the other hand, according to the literature (16, 17), the volume fraction of the solvent mixture in the saturated solution has been calculated by means of the expression, where, V 1 is the molar volume of the solvent (calculated for solvent mixtures as V V mix = ΣV1iÏi, assuming additive volumes). Nevertheless, it is well known that the mixing volumes are not additives in those mixtures with strong presence of hydrogen bonding and great differences in molar volumes among their components. For this reason, the experimental volume fractions were used in this investigation for all the calculations involved (Table 1).

Ultimately, the activity coefficients of IMC as decimal logarithms are also presented in Table 1. These values were calculated from experimental solubility values (Table 1) and ideal solubility at 298.15 K (X 2 = 7.123 x 10 -3 ). In all cases, γ 2 values were greater than unit because the experimental solubilities are lower than the ideal one. On the other hand, the parameters A, K, and W are presented in Table 2. In order to calculate the W parameter the experimental solubility parameter of IMC obtained in ethyl acetate + ethanol mixtures (20.86 MPa 1/2 ) was used. This δ 2 value is the same of the solvent mixture where the greatest drug solubility was found (15).
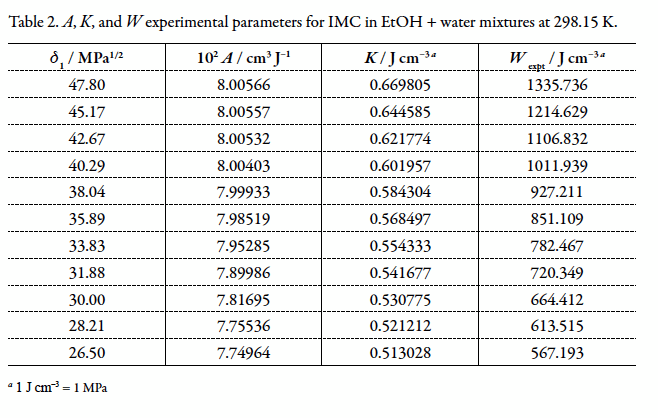
As has been already indicated, the W parameter accounts for the deviations presented by real solutions with respect to regular solutions. These deviations are mainly due to specific interactions such as hydrogen bonding. IMC (Fig. 1) and both solvents studied can establish these interactions, as hydrogen donors or acceptors because of their polar moieties, in particular due to -OH groups. Figure 3 shows that the variation of the W parameter with respect to the solubility parameter of solvent mixtures, presents deviation from linear behavior. W values were adjusted to regular polynomials in orders from 1 to 5 (equation 6) and their coefficients and statistical parameters are presented in the Table 3 (the empirical regressions were obtained by using MS Excel® and TableCurve 2D v5.01). The W values calculated by using the respective regular polynomials are presented inTable 4. It is well clear that these values depend on the model used in the W back-calculation. Similar behaviors have been reported in the literature for several other compounds (5-7, 10-17).



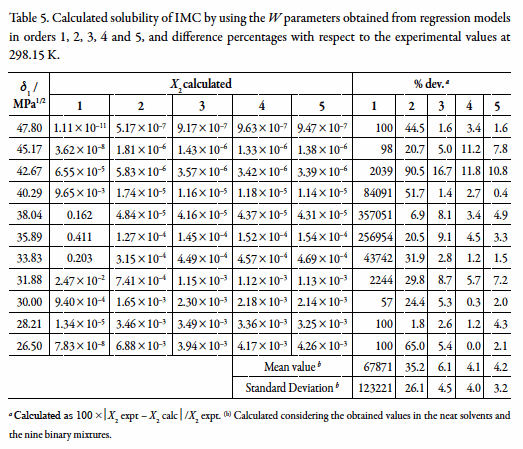
According to Table 5 it follows that, as more complex the polynomial used is, better the agreement found between experimental and calculated solubility is. This fact is confirmed based on the mean deviation percentages (4.1 % and 4.2 %, for orders 4 and 5, respectively). In similar way to that found in other similar investigations (4-11), in this case, the most important increment in concordance is obtained passing from order 1 to order 2 (from 67871 to 35.2 % as mean value), although significant increment is also obtained form order 2 to order 3 (from 35.2 to 6.1% as mean value). Thereby, in the following calculations the model with lowest general deviation was used (order 4, Table 3).
An important consideration about the usefulness of the EHSA method is the one referent to justify the complex calculations involving any other variables of the considered system (Equation 4, Tables 1 to 4), instead of the simple empiric regression of the experimental solubility as a function of the solvent mixtures´ solubility parameters (Table 1 , Fig. 2). For this reason, in the Table 6 the experimental solubilities are confronted to those calculated directly by using a regular polynomial in order 4 of log X 2 as a function of δ 1 values (Equation 9, with determination coefficient r 2 = 0.999 and fitting standard uncertainty = 0.033) and also to those calculated involving the W parameters obtained from equation 6 adjusted to order 4 (Table 3). The respective difference percentages are also presented in Table 6.
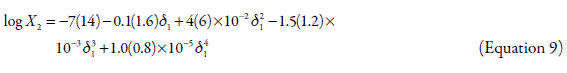
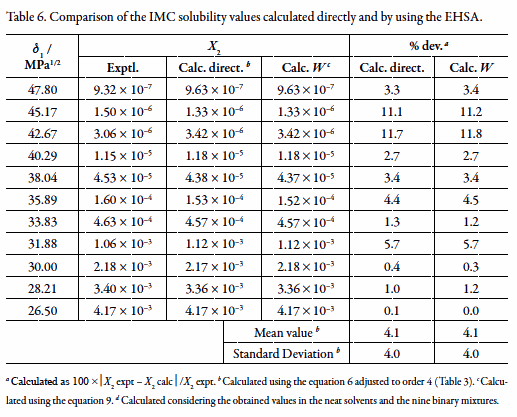
Based on mean deviation percentages presented in Table 6 (4.1% and 4.1% for direct calculation and EHSA method, respectively) it follows that non-significant differences are found between the values obtained by using both methods. In similar way with that found for naproxen and ketoprofen (other analgesic drugs) in the same cosolvent system (13, 14), and for IMC in ethyl acetate + ethanol mixtures (15), the present results would be showing non-significant usefulness of EHSA method for practical purposes. The last point exposed would be a big controversial subject considering that EHSA method implies additional experimentation including density determinations and thermal characterization of the solid-liquid equilibrium for the solid compound. Nevertheless, it is necessary keep in mind that EHSA method considers the drug solubility from a systematic physicochemical point of view. Moreover, it is just necessary to found an effective method to calculate the Walker K parameter in order to calculate the W term according to the expression 2Kδ1δ2 , because the δ1 and δ2 terms would be known, and thus, the drug experimental solubility could be calculated in any mixture in particular.Table 5 summarizes the solubility values obtained by using the W values obtained by back-calculation from the polynomial models presented in Table 4. Because we are searching the best adjust, the first criterion used to define the polynomial order of W as function of δ 1 was the fitting standard uncertainties obtained, whose values were as follows, 22.0, 1.23, 0.247, 0.208, and 0.208 (Table 3), for orders one to five, respectively. As another comparison criterion,Table 5 also summarizes the percentages of difference between IMC experimental solubility and those calculated by using EHSA.
CONCLUSION
In this investigation the EHSA method has been adequately used to study the solubility of IMC in EtOH + water mixtures by using experimental values of molar volume and Hildebrand solubility parameter of this analgesic drug. In particular, a good predictive character has been found by using a regular polynomial in order four of the interaction parameter W as a function of the solubility parameter of solvent mixtures free of solute. Form a practical viewpoint EtOH + water binary mixtures were thus constituted in good cosolvent systems for IMC, especially at higher fractions of alcohol, by generate a decrease on polarity of the medium.
ACKNOWLEDGEMENTS
The authors thank to the Department of Pharmacy of the Universidad Nacional de Colombia for facilitating the equipments and installations used in this investigation.
REFERENCES
1. S. Budavari, M.J. O´Neil, A. Smith, P.E. Heckelman, J.R. Obenchain Jr., J.A.R. Gallipeau, M.A. D´Arecea, "The Merck Index, An Encyclopedia of Chemicals, Drugs, and Biologicals", 13th ed., Merck & Co., Inc., Whitehouse Station, NJ, 2001, p. 892.
2. R.B. Raffa, Analgesic, antipyretic, and anti-inflammator y drugs , in : "Remington: The Science and Practice of Pharmacy". 21 st ed., edited by A. Gennaro, Lippincott Williams & Wilkins, Philadelphia, 2005, pp. 1524-1542.
3. L.R. Lépori, A. Cohen, H.E. Castagneto, S. Benítez, E.C. Peyrás, "P.R. Vademecum", 4th ed., Licitelco S.A., Bogotá, 2005, p. 358.
4. F. Jiménez, F. Martínez, Una estrategia para la selección sistemática de vehículos en el diseño de forma farmacéuticas líquidas homogéneas, Rev. Colomb. Cienc. Quím. Farm., 24(1), 19-23 (1995).
5. A. Martin, J. Newburger, A. Adjei, Extended Hildebrand approach: solubility of caffeine in dioxane-water mixtures, J. Pharm. Sci., 69(6), 659-661 (1980).
6. A. Martin, P.L. Wu, Extended Hildebrand solubility approach: p-Hydroxi-benzoic acid in mixtures of dioxane and water, J. Pharm. Sci., 72(6), 587-592 (1981).
7. A. Martin, M.J. Miralles, Extended Hildebrand solubility approach: Solubility of tolbutamide, acetohexamide, and sulfisomidine in binary solvent mixtures, J. Pharm. Sci., 71(4), 439-442 (1982).
8. J.T. Rubino, Cosolvents and cosolvency, in "Encyclopedia of Pharmaceutical Technology", Vol 3, edited by J. Swarbrick, J.C. Boylan, Marcel Dekker, Inc., New York, 1988. pp. 375-398.
9. S.H. Yalkowsky, T.J. Roseman, Solubilization of drugs by cosolvents, in "Techniques of Solubilization of Drugs", edited by S.H. Yalkowsky, Marcel Dekker, Inc., New York, 1981, pp. 91-134.
10. F. Martínez, Aplicación del método extendido de Hildebrand al estudio de la solubilidad del acetaminofén en mezclas etanol-propilenoglicol, Acta Farm. Bonaerense, 24(2), 215-224 (2005).
11. F. Martínez, Utilidad del método extendido de Hildebrand en el estudio de la solubilidad del acetaminofén en mezclas agua-propilenoglicol, Rev. Acad. Colomb. Cienc., 29(112), 429-438 (2005).
12. D.P. Pacheco, Y.J. Manrique, E.F. Vargas, H.J. Barbosa, F. Martínez, Validez del método extendido de Hildebrand en la predicción de las solubilidades de ibuprofén y naproxén en mezclas propilenoglicol-etanol, Rev. Colomb. Quím., 36(1), 55-72 (2007).
13. D.M. Aragón, D.P. Pacheco, M.A. Ruidiaz, A.D. Sosnik, F. Martínez, Método extendido de Hildebrand en la predicción de la solubilidad de naproxeno en mezclas cosolventes etanol + agua, Vitae, Rev. Fac. Quím. Farm., 15(1), 113-122 (2008).
14. M. Gantiva, F. Martínez, Método extendido de Hildebrand en la predicción de la solubilidad del ketoprofeno en mezclas cosolventes etanol + agua, Quím. Nova, 33(2), 370-376 (2010).
15. M.A. Ruidiaz, F. Martínez, Método extendido de Hildebrand en la estimación de la solubilidad de la indometacina en mezclas acetato de etilo + etanol, Rev. Colomb. Quím., 38(2), 235-247 (2009).
16. A. Martin, P. Bustamante, El parámetro de solubilidad en las ciencias farmacéuticas, Anal. Real Acad. Farm., 55(2), 175-202 (1989).
17. A. Martin, P. Bustamante, A.H.C. Chun, "Physical Chemical Principles in the Pharmaceutical Sciences", 4 th edition, Lea & Febiger, Philadelphia, 1993.
18. J.H. Hildebrand, J.M. Prausnitz, R.L. Scott, "Regular and Related Solutions",Van Nostrand Reinhold, New York, 1970.
19. BP 1988, The British Pharmacopoeia, Vol. I, Her Majesty´s Stationery Office, London, 1988, p. 310.
20. J.M. Resa, C. González, J.M. Goenaga, M. Iglesias, Temperature dependence of excess molar volumes of ethanol + water + ethyl acetate, J. Solution Chem., 33(2), 169-198 (2004).
21. R. Belda, J.V. Herraez, O. Diez, Rheological study and thermodynamic analysis of the binary system (water/ethanol): influence of concentration, Phys. Chem. Liq., 42(5), 467-476 (2004).
22. J.A. Jiménez, F. Martínez, Thermodynamic study of the solubility of acetaminophen in propylene glycol + water cosolvent mixtures, J. Braz. Chem. Soc., 17(1), 125-134 (2006).
23. J. Jiménez, J. Manrique, F. Martínez, Effect of temperature on some volumetric properties for ethanol + water mixtures, Rev. Colomb. Cienc. Quím. Farm., 33(2), 145-155 (2004).
24. A. Yurquina, M.E. Manzur, M.A.A. Molina, R. Manzo, Requerimiento dieléctrico y parámetro de solubilidad de nipagín y nipasol en el sistema agua-etanol, Acta Farm. Bonaerense, 19(1), 49-52 (2000).
25. A.N. Paruta, B.J. Sciarrone, N.G. Lordi, Correlation between solubility parameters and dielectric constants, J. Pharm. Sci., 51(7), 704-705 (1962).
26. A. Forster, J. Hempenstall, I. Tucker, T. Rades, Selection of excipients for melt extrusion with two poorly water-soluble drugs by solubility parameter calculation and thermal analysis, Int. J. Pharm., 226(1, 2), 147-161 (2001).
27. R.F. Fedors, A method for estimating both the solubility parameters and molar volumes of liquids, Polymer Eng. Sci., 14(2), 147-154 (1974).
How to Cite
APA
ACM
ACS
ABNT
Chicago
Harvard
IEEE
MLA
Turabian
Vancouver
Download Citation
Article abstract page views
Downloads
License
Copyright (c) 2010 Revista Colombiana de Ciencias Químico-Farmacéuticas

This work is licensed under a Creative Commons Attribution 4.0 International License.
The Department of Pharmacy of the Faculty of Sciences of the National University of Colombia authorizes the photocopy of articles and texts for academic or internal purposes of the institutions, citing the source. The ideas issued by the authors are the express responsibility of these and it does not necessarily reflect the views of this journal.
The entire contents of this journal, except when is identified, are subject to a Creative Commons Attribution License 4.0 adopted by Colombia. Consult the regulation: http://co.creativecommons.org/?page_id=13













