Published
The Formalism of Chemical Thermodynamics Applied to an Oscillatory Multistep Chemical System
El formalismo de la termodinámica química aplicado a un sistema químico oscilatorio con múltiples etapas
DOI:
https://doi.org/10.15446/ing.investig.108212Keywords:
chemical process simulation, chemical equilibrium, the Oregonator, Belousov-Zhabotinsky reaction, oscillating reactions (en)simulación de procesos químicos, equilibrio químico, el Oregonador, reacción de Belousov-Zhabotinsky, reacciones oscilantes (es)
Downloads
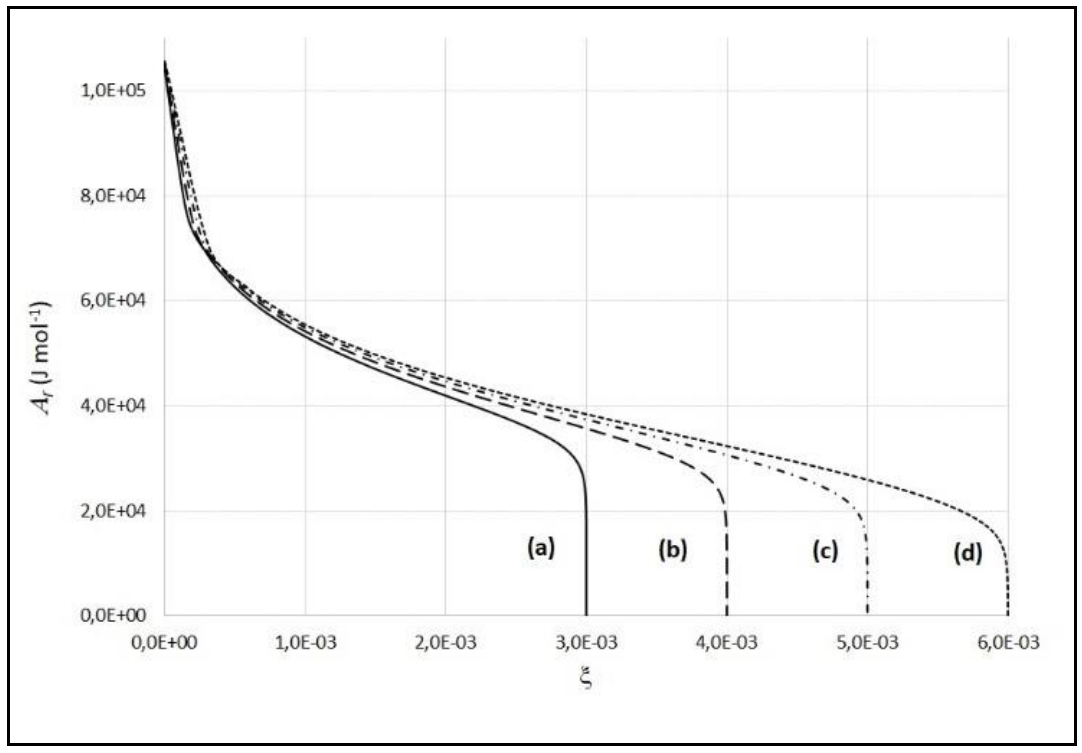
The thermodynamic optimization of a process focuses on consumption, production, and efficient use of energy. The unsteady-state nature of batch reactor processing requires describing the set of processes’ dynamic behavior for energy optimization. This work aims to apply the formalism of chemical thermodynamics to a multistep chemical system in a batch reactor, aiming for a dynamic description of its evolution to the equilibrium state. As the system of study, we selected a mathematical model called the Oregonator, derived from the mechanism of the oscillating Belousov-Zhabotinsky reaction. In the methodology, we used the reaction quotient to evaluate the Gibbs function, the thermodynamic affinity, and the entropy generation as a function of the reaction extent. The results show that the overall reaction fulfills the thermodynamic fundamentals of chemical equilibrium, despite having a non-stoichiometric coefficient. However, the multistep coupled reaction system does not allow verifying compliance with the thermodynamic foundations of chemical equilibrium. We conclude that it is necessary to improve thermodynamic formalism to describe multistep chemical processes as a function of a global reaction extent variable. In this scenario, the entropy production rate emerges as a promising quantity.
La optimización termodinámica de un proceso se enfoca en el consumo, la producción y el uso eficiente de la energía. La naturaleza de estado no estacionario del procesamiento en reactores tipo batch requiere describir el comportamiento dinámico del conjunto de procesos en términos de optimización de energía. Este trabajo tiene como objetivo aplicar el formalismo de la termodinámica química a un sistema químico de múltiples etapas en un reactor tipo batch, buscando obtener una descripción dinámica de su evolución hacia el estado de equilibrio. Como sistema de estudio, seleccionamos el modelo matemático conocido como el Oregonator, que se deriva del mecanismo de la reacción oscilante de Belousov-Zhabotinsky. En la metodología, utilizamos el cociente de reacción para evaluar la función de Gibbs, la afinidad termodinámica y la generación de entropía en función del grado de avance de la reacción. Los resultados muestran que la reacción global cumple con los fundamentos termodinámicos del equilibrio químico, a pesar de tener un coeficiente no estequiométrico. Sin embargo, el sistema de reacción con múltiples pasos acoplados no permite verificar el cumplimiento de los fundamentos termodinámicos del equilibrio químico. Concluimos que es necesario mejorar el formalismo termodinámico para describir los procesos químicos de múltiples pasos en función de una variable global de avance de la reacción. En este escenario, la velocidad de generación de entropía surge como una cantidad prometedora.
References
Arango-Restrepo, A., Barragán, D., and Rubi, J. M. (2020). Modelling non-equilibrium self-assembly from dissipa-tion. Molecular Physics, 118(9-10), e1761036. https://doi.org/10.1080/00268976.2020.1761036 DOI: https://doi.org/10.1080/00268976.2020.1761036
Banerjee, A. C. (1995). Teaching chemical equilibrium and thermodynamics in undergraduate general chemistry classes. Journal of Chemical Education, 72(10), 879. https://doi.org/10.1021/ed072p879 DOI: https://doi.org/10.1021/ed072p879
Barragán, D., Ágreda, J., and Parra, W. (2015). Entropy pro-duction in the Oregonator model perturbed in a calo-rimeter with a chemical pulse. Journal of Thermal Analysis and Calorimetry, 119, 705-713. https://doi.org/10.1007/s10973-014-4129-0 DOI: https://doi.org/10.1007/s10973-014-4129-0
Barragán, D., and Montoya, J. P. (2021). Producción de en-tropía en un modelo autocatalítico no-isotérmico perturbado con pulsos de analito. Revista de la Fa-cultad de Ciencias, 10(2), 9-27. https://doi.org/10.15446/rev.fac.cienc.v10n2.83839 DOI: https://doi.org/10.15446/rev.fac.cienc.v10n2.83839
Beretta, G. P., and Gyftopoulos, E. P. (2015). What is a chemi-cal equilibrium state. Journal of Energy Resources Technology, 137(2), 4. https://dx.doi.org/10.1115/1.4026384 DOI: https://doi.org/10.1115/1.4026384
de Oliveira, L. P., Hudebine, D., Guillaume, D., and Verstraete, J. J. (2016). A review of kinetic modeling methodol-ogies for complex processes. Oil & Gas Science and Technology–Revue d’IFP energies nouvelles, 71(3), 45. https://doi.org/10.2516/ogst/2016011 DOI: https://doi.org/10.2516/ogst/2016011
Dutt, A. K. (1985). Instabilities and oscillations in ‘‘The reversible oregonator model’’: A thermodynamic approach to calculate the excess entropy production (δ m P) and the corresponding antisymmetric function (δ m Π). The Journal of Chemical Physics, 82(10), 4678-4682. http://dx.doi.org/10.1063/1.448972 DOI: https://doi.org/10.1063/1.448972
Epstein, I. R., and Pojman, J. A. (1998). An introduction to nonlinear chemical dynamics: oscillations, waves, pat-terns, and chaos, Oxford University Press. DOI: https://doi.org/10.1093/oso/9780195096705.001.0001
Eu, B. C., and Al-Ghoul, M. (2018). Chemical thermodynamics: Reversible and irreversible thermodynamics. World Sci-entific. DOI: https://doi.org/10.1142/10599
Field, R. J., Koros, E., and Noyes, R. M. (1972). Oscillations in chemical systems. II. Thorough analysis of temporal os-cillation in the bromate-cerium-malonic acid system. Journal of the American Chemical Society, 94(25), 8649-8664. https://doi.org/10.1021/ja00780a001 DOI: https://doi.org/10.1021/ja00780a001
Field, R. J., and Noyes, R. M. (1974). Oscillations in chemical systems. IV. Limit cycle behavior in a model of a real chemical reaction. The Journal of Chemical Physics, 60(5), 1877-1884. https://doi.org/10.1063/1.1681288 DOI: https://doi.org/10.1063/1.1681288
Haseli, Y. (2019). Criteria for chemical equilibrium with applica-tion to methane steam reforming. International Journal of Hydrogen Energy, 44(12), 5766-5772. https://doi.org/10.1016/j.ijhydene.2019.01.130 DOI: https://doi.org/10.1016/j.ijhydene.2019.01.130
Heimburg, T. (2017). Linear nonequilibrium thermodynamics of reversible periodic processes and chemical oscillations. Physical Chemistry Chemical Physics, 19(26), 17331-17341. https://doi.org/10.1039/C7CP02189E DOI: https://doi.org/10.1039/C7CP02189E
Heimburg, T. (2021). Damped physical oscillators, temperature and chemical clocks. arXiv preprint. https://doi.org/10.48550/arXiv.2112.03083
Honig, J. M. (2020). Thermodynamics: Principles characterizing physical and chemical processes. Academic Press.
Kangas, P. (2015). Modelling the super-equilibria in thermal biomass conversion: Applications and limitations of the constrained free energy method [Technical Re-port]. VTT Technical Research Centre of Finland LTD. https://publications.vtt.fi/pdf/science/2015/S92.pdf
Kangas, P., Vázquez, F. V., Savolainen, J., Pajarre, R., and Koukkari, P. (2017). Thermodynamic modelling of the methanation process with affinity constraints. Fuel, 197, 217-225. https://doi.org/10.1016/j.fuel.2017.02.029 DOI: https://doi.org/10.1016/j.fuel.2017.02.029
Kiprijanov, K. S. (2016). Chaos and beauty in a beaker: The early history of the Belousov‐Zhabotinsky reaction. An-nalen der Physik, 528(3-4), 233-237. https://doi.org/10.1002/andp.201600025 DOI: https://doi.org/10.1002/andp.201600025
Kondepudi, D., and Prigogine, I. (2014). Modern thermody-namics: From heat engines to dissipative structures, John Wiley & Sons. DOI: https://doi.org/10.1002/9781118698723
Koukkari, P., Pajarre, R., and Kangas, P. (2018). Thermodynam-ic affinity in constrained free-energy systems. Monatshefte für Chemie-Chemical Monthly, 149, 381-394. https://doi.org/10.1007/s00706-017-2095-5 DOI: https://doi.org/10.1007/s00706-017-2095-5
Koukkari, P., and Pajarre, R. (2021). Phase diagrams with the driving force and extent of reaction as axis variables. Calphad, 74, 102290. https://doi.org/10.1016/j.calphad.2021.102290 DOI: https://doi.org/10.1016/j.calphad.2021.102290
Marin, G. B., Yablonsky, G. S., and Constales, D. (2019). Kinet-ics of chemical reactions: decoding complexity. John Wiley & Sons. DOI: https://doi.org/10.1002/9783527808397
Martínez-Grau, M., Solaz-Portolés, J. J., and Sanjosé, V. (2014). Propuesta de un test para determinar el conocimiento conceptual de estudiantes universitarios sobre la cons-tante de equilibrio químico y su aplicación en estu-diantes españoles. Química Nova, 37, 740-744. http://dx.doi.org/10.5935/0100-4042.20140118 DOI: https://doi.org/10.5935/0100-4042.20140118
Nieto-Villar, J. M. (2020). Una mirada a los sistemas complejos desde la termodinámica. Suplemento de la Revista Mexicana de Física, 1(4), 17-24. https://doi.org/10.31349/SuplRevMexFis.1.4.17 DOI: https://doi.org/10.31349/SuplRevMexFis.1.4.17
Ojelade, O. A., and Zaman, S. F. (2021). Ammonia decompo-sition for hydrogen production: a thermodynamic study. Chemical Papers, 75, 57-65. https://doi.org/10.1007/s11696-020-01278-z DOI: https://doi.org/10.1007/s11696-020-01278-z
Patiño-Sierra, D. F., and Barragán, D. (2022). Un método for-mal para la armonización conceptual del equilibrio químico. Revista de la Facultad de Ciencias, 11(2), 148-161. https://doi.org/10.15446/rev.fac.cienc.v11n2.99977 DOI: https://doi.org/10.15446/rev.fac.cienc.v11n2.99977
Prausnitz, J. M., Lichtenthaler, R. N., and de Azevedo, E. G. (1998). Molecular thermodynamics of fluid-phase equi-libria. Pearson Education.
Prigogine, I. (1961). Introduction to irreversible thermodynam-ics. Interscience.
Quílez‐Pardo, J., and Solaz‐Portolés, J. J. (1995). Students' and teachers' misapplication of Le Chatelier's principle: Im-plications for the teaching of chemical equilibrium. Journal of Research in Science teaching, 32(9), 939-957. https://doi.org/10.1002/tea.3660320906 DOI: https://doi.org/10.1002/tea.3660320906
Rogers, F., Huddle, P. A., and White, M. W. (2000). The com-plexity of teaching and learning chemical equilibrium. Journal of Chemical Education, 76(4), 554. https://doi.org/10.1021/ed076p554 DOI: https://doi.org/10.1021/ed076p554
Rosenberg, R. M., and Klotz, I. M. (2008). Chemical thermody-namics: basic concepts and methods. Wiley.
Saunders, N., and Miodownik, A. P. (Eds.) (1998). CALPHAD (calculation of phase diagrams): A comprehensive guide. Elsevier.
Solaz-Portolés, J. J. (2011). Variation of extent of reaction in closed chemical equilibrium when changing the tem-perature at constant volume. Química Nova, 34, 710-713. [https://doi.org/10.1590/S0100-40422011000400028] DOI: https://doi.org/10.1590/S0100-40422011000400028
Tosun, I. (2021). The thermodynamics of phase and reaction equilibria. Elsevier. DOI: https://doi.org/10.1016/B978-0-12-820530-3.00015-5
Tyson, J. J. (1981). On scaling the oregonator equations. In C. Vidal and Pacault (Eds.), Nonlinear Phenomena in Chemical Dynamics (pp. 222-227). Springer. https://doi.org/10.1007/978-3-642-81778-6_33 DOI: https://doi.org/10.1007/978-3-642-81778-6_33
Tyson, J. J. (1982). Scaling and reducing the Field-Koros-Noyes mechanism of the Belousov-Zhabotinskii reaction. The Journal of Physical Chemistry, 86(15), 3006-3012. https://doi.org/10.1021/j100212a039 DOI: https://doi.org/10.1021/j100212a039
Wang, C., Chen, L., Xia, S., and Sun, F. (2016). Maximum pro-duction rate optimization for sulphuric acid decompo-sition process in tubular plug-flow reactor. Energy, 99, 152-158. https://doi.org/10.1016/j.energy.2016.01.040 DOI: https://doi.org/10.1016/j.energy.2016.01.040
How to Cite
APA
ACM
ACS
ABNT
Chicago
Harvard
IEEE
MLA
Turabian
Vancouver
Download Citation
CrossRef Cited-by
Dimensions
PlumX
Article abstract page views
Downloads
License
Copyright (c) 2024 Jean P. Montoya, Alexander Contreras-Payares, Daniel Barragán

This work is licensed under a Creative Commons Attribution 4.0 International License.
The authors or holders of the copyright for each article hereby confer exclusive, limited and free authorization on the Universidad Nacional de Colombia's journal Ingeniería e Investigación concerning the aforementioned article which, once it has been evaluated and approved, will be submitted for publication, in line with the following items:
1. The version which has been corrected according to the evaluators' suggestions will be remitted and it will be made clear whether the aforementioned article is an unedited document regarding which the rights to be authorized are held and total responsibility will be assumed by the authors for the content of the work being submitted to Ingeniería e Investigación, the Universidad Nacional de Colombia and third-parties;
2. The authorization conferred on the journal will come into force from the date on which it is included in the respective volume and issue of Ingeniería e Investigación in the Open Journal Systems and on the journal's main page (https://revistas.unal.edu.co/index.php/ingeinv), as well as in different databases and indices in which the publication is indexed;
3. The authors authorize the Universidad Nacional de Colombia's journal Ingeniería e Investigación to publish the document in whatever required format (printed, digital, electronic or whatsoever known or yet to be discovered form) and authorize Ingeniería e Investigación to include the work in any indices and/or search engines deemed necessary for promoting its diffusion;
4. The authors accept that such authorization is given free of charge and they, therefore, waive any right to receive remuneration from the publication, distribution, public communication and any use whatsoever referred to in the terms of this authorization.



























